Odd Numbers
Odd numbers are numbers that cannot be arranged in pairs. In other words, all numbers except the multiples of 2 are odd numbers. Let us learn more about odd numbers, the definition of odd numbers, the odd numbers list and some examples of odd numbers in this article.
| 1. | What are Odd Numbers? |
| 2. | List of Odd Numbers |
| 3. | Properties of Odd Numbers |
| 4. | Types of Odd Numbers |
| 5. | FAQs on Odd Numbers |
What are Odd Numbers?
Odd numbers are those numbers that cannot be divided into two parts equally. In other words, odd numbers are positive integers that cannot be categorized into groups of two.
Meaning of Odd Numbers
Let us understand the meaning of odd numbers with the help of an example. If we observe numbers like, 1, 3, 5, 7, and so on, we see that these numbers cannot be paired. Let us visualize it using an example of shoes and cherries. Observe the figure given below in order to understand how the pairing of these numbers works. We have sets of shoes in counts of 1, 3, 5, and 7. On the other hand, we have cherries in pairs of 2, 4, 6, and 8.
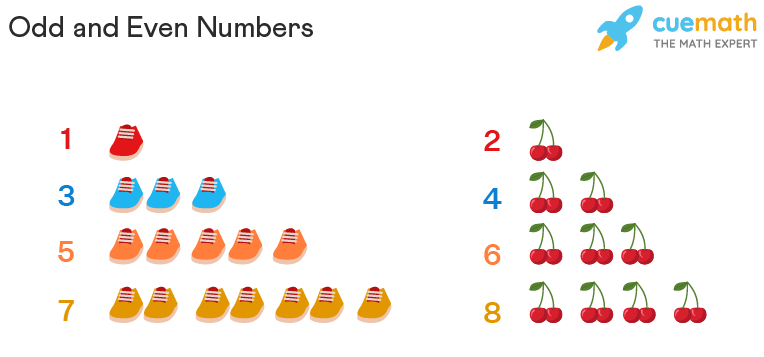
It is to be noted here that the shoes, which show odd numbers, do not form complete pairs. One shoe among all remains unpaired. On the contrary, cherries that show even numbers are those numbers that can be divided into two parts equally and so they form complete pairs. For example, 4 cherries can be divided into 2 pairs of 2, 8 cherries can be divided into 4 pairs of 2, and so on.
Therefore, we can understand that odd numbers cannot be paired.
List of Odd Numbers
Let us have a look at the list of all the odd numbers from 1 to 200 and try to apply the knowledge we have learned here so far. Note that none of the numbers given here are multiples of 2. It should also be noted that out of the first 200 numbers, only 100 numbers are odd numbers. Have a look at the list of the odd numbers from 1 to 200 given here.
| 1 | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 |
| 21 | 23 | 25 | 27 | 29 | 31 | 33 | 35 | 37 | 39 |
| 41 | 43 | 45 | 47 | 49 | 51 | 53 | 55 | 57 | 59 |
| 61 | 63 | 65 | 67 | 69 | 71 | 73 | 75 | 77 | 79 |
| 81 | 83 | 85 | 87 | 89 | 91 | 93 | 95 | 97 | 99 |
| 101 | 103 | 105 | 107 | 109 | 111 | 113 | 115 | 117 | 119 |
| 121 | 123 | 125 | 127 | 129 | 131 | 133 | 135 | 137 | 139 |
| 141 | 143 | 145 | 147 | 149 | 151 | 153 | 155 | 157 | 159 |
| 161 | 163 | 165 | 167 | 169 | 171 | 173 | 175 | 177 | 179 |
| 181 | 183 | 185 | 187 | 189 | 191 | 193 | 195 | 197 | 199 |
The definition that we have learned above is applied in this table and it eases our work, doesn't it? Observe the given table and try to notice some similarities between all these numbers given above.
- Did you notice a pattern in the above odd numbers list?
- In the odd numbers list, the digit at the ones place always remains 1, 3, 5, 7, or 9.
Properties of Odd Numbers
The following points list the properties of odd numbers. Each of these properties can be explained in a detailed way as given below.
- Addition of Odd Numbers: The addition of two odd numbers always gives an even number, i.e., the sum of two odd numbers is always an even number. For example, 3 (odd) + 5 (odd) = 8 (even).
- Subtraction of Odd Numbers: Subtraction of two odd numbers always results in an even number. For example, 7 (odd) - 1 (odd) = 6 (even).
- Multiplication of Odd Numbers: Multiplication of two odd numbers always gives an odd number. For example, 3 (odd) × 7 (odd) = 21 (odd).
- Division Of Odd Numbers: Division of two odd numbers always gives an odd number. For example, 33 (odd) ÷ 11 (odd) = 3 (odd).
Let us summarize these properties of odd numbers using the table given below.
| Arithmetic Operation | Result |
|---|---|
| Odd number + Odd number | Even number |
| Odd number - Odd number | Even number |
| Odd number × Odd number | Odd number |
| Odd number ÷ Odd number | Odd number |
Types of Odd Numbers
Odd numbers are a list of all the numbers that are not the multiples of 2. So this seems like a vast set of numbers. So we can have many types of odd numbers starting from whether the odd numbers have factors or not, what is the difference between two odd numbers, what is their position on the number line, etc.
Consecutive Odd Numbers
Consecutive odd numbers are those odd numbers that are listed in sequence of their order. For example, if n is an odd number, then the numbers n and n + 2 are grouped under the category of consecutive odd numbers. They always have a difference of 2 between them and are consecutive in nature, hence the name consecutive odd numbers. For example 3 and 5, 11 and 13, 25 and 27, 37 and 39, 49 and 51, and so on.
Composite Odd Numbers
Composite odd numbers are those odd numbers that are not prime numbers. These types of odd numbers are formed by the product of two smaller positive odd integers. The composite odd numbers from 1 to 100 are 9, 15, 21, 25, 27, 33, 35, 39, 45, 49, 51, 55, 57, 63, 65, 69, 75, 77, 81, 85, 87, 91, 93, 95, and 99.
Smallest Odd Number
The smallest odd number is 1 because odd numbers start from 1 and are listed as, 1, 3, 5, 7, 9, 11 and so on.
First 10 Odd Numbers
The first ten odd numbers are 1, 3, 5, 7, 9, 11, 13, 15, 17, and 19.
Smallest Odd Composite Number
The smallest odd composite number is 9. If we start looking at the smallest composite number it is 4, but that is an even number. The next composite number is 6 which is again an even number. The same goes for the next composite number which is 8. Now, the next composite number is 9 which is an odd number. So, this brings us to the smallest odd composite number which is 9.
Odd Prime Numbers
Odd prime numbers means those prime numbers which are odd numbers. Interestingly, all prime numbers except 2 are odd numbers.
Odd Natural Numbers
Odd natural numbers mean natural numbers that are odd numbers. We know that natural numbers are positive counting numbers that start from 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, and go on till infinity. Among these if we select the odd numbers, we get 1, 3, 5, 7, 9, and so on.
Tips and Tricks on Odd Numbers
Given below is a list of a few tips and tricks on odd numbers. These will help you in remembering the concepts faster.
- The sum of two odd numbers is always an even number.
- An easy method to differentiate whether a number is odd or even is to divide it by 2.
- If the number is not divisible by 2 completely, it will leave a remainder of 1, which indicates that the number is an odd number and cannot be divided into 2 parts evenly.
- If the number is divisible by 2 completely, it will leave a remainder 0, which indicates that it is an even number and it can be divided into 2 parts evenly.
- Odd numbers always have 1, 3, 5, 7, or 9 in their units place. Even numbers always have 0, 2, 4, 6, or 8 in their units place.
- Even Numbers
- Real Numbers
- Natural Numbers
- Whole Numbers
- Rational Numbers
- Irrational Numbers
- Counting Numbers
- Cardinal Numbers
- Odd Numbers 1 to 1000
- Sum of Odd Numbers
- Even and Odd Numbers Worksheets
Cuemath is one of the world's leading math learning platforms that offers LIVE 1-to-1 online math classes for grades K-12. Our mission is to transform the way children learn math, to help them excel in school and competitive exams. Our expert tutors conduct 2 or more live classes per week, at a pace that matches the child's learning needs.
Examples of Odd Numbers
-
Example 1: Determine whether 135 is an odd number or not.
Solution:
We can find this out using any of the given two methods:
Method 1: Check the digit on the units place. The units place of the number is 5, which is an odd number. This shows that the given number is an odd number. Therefore, 135 is an odd number.
Method 2: We need to check the divisibility of the number by 2. On dividing the given number 135 by 2, we get the remainder as 1. This proves that 135 is an odd number.
Therefore, we know that the number 135 is an odd number.
-
Example 2: Answer the following questions with reference to odd numbers:
a.) 1 is odd or even?
b.) Which is the smallest 4 digit odd number?
c.) What is the smallest odd composite number?
d.) What is the sum of any two odd numbers?
e.) Is 2 an odd number?
Solution:
a.) 1 is an odd number.
b.) The smallest 4 digit odd number is 1001.
c.) The smallest odd composite number is 9.
d.) The sum of any two odd numbers is always an even number.
e.) No, 2 is not an odd number, it is an even number.
-
Example 3: State true or false with respect to odd numbers.
a.) The sum of two odd numbers is always an even number.
b.) The smallest odd number is 5.
c.) All prime numbers are odd numbers.
d.) 9 is an odd number.
Solution:
a.) True, the sum of two odd numbers is always an even number.
b.) False, the smallest odd number is 1.
c.) False, all prime numbers are not odd numbers, because 2 is a prime number which is even. It can be said that except 2, all prime numbers are odd.
d.) True, 9 is an odd number.

FAQs on Odd Numbers
What are Odd Numbers in Math?
In math, odd numbers are those numbers that are not the multiples of 2. For example, 3, 5, 7, 9, and so on. Odd numbers cannot be arranged in pairs which means that they cannot be divided into two parts equally.
What are the Odd Numbers From 1 to 100?
The list of odd numbers from 1 to 100 is: 1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25, 27, 29, 31, 33, 35, 37, 39, 41, 43, 45, 47, 49, 51, 53, 55, 57, 59, 61, 63, 65, 67, 69, 71, 73, 75, 77, 79, 81, 83, 85, 87, 89, 91, 93, 95, 97, 99.
What is the Difference Between an Odd Number and an Even Number?
The numbers which can be divided into two parts evenly are called even numbers. For example, 4, 8, 82, and so on are even numbers. Whereas odd numbers cannot be divided into equal pairs and are not divisible by 2. For example, 7, 91, 63, and so on are odd numbers.
What are Consecutive Odd Numbers?
Two consecutive odd numbers are those numbers that are odd and have a difference of 2 between them. For example 3 and 5, 11 and 13, and so on.
Is 0 an Even or Odd Number?
0 is an even number because it is attached with the multiples of 2. For example, 10, 20, 30 40, and so on. Therefore, it is not an odd number.
What is the Mean of the First Five Odd Numbers?
In order to find the mean of the first five odd numbers we need to follow the steps listed below:
- Step 1: List the first five odd numbers, they are 1, 3, 5, 7, and 9.
- Step 2: Calculate the sum → 1 + 3 + 5 + 7 + 9 = 25.
- Step 3: Apply the mean formula = Mean of any n numbers = (Sum of n numbers) ÷ n = 25/5 = 5.
Therefore, the mean of the first five odd numbers is 5.
How Many Odd Numbers are there Between 36 and 53?
The list of odd numbers that lie between 36 and 53 are 37, 39, 41, 43, 45, 47, 49, and 51. Hence, there are a total of 8 odd numbers between 36 and 53.
Is Number 1 Odd or Even?
1 is an odd number because odd numbers are not divisible by 2 completely. For example, 3, 5, and 7 are a few odd numbers that cannot be divided equally into groups of 2..
Which Odd Number Comes After 499?
The odd number that comes after 499 is 501. The number that comes after 499 is 500 which is an even number. So, 501 is the next odd number after 499.
What is the Odd Numbers Formula?
The odd numbers formula is expressed as 2n ± 1, where, n ∈ W (whole numbers). We know that an odd number is always expressed as 1 less or more than an even number. An even number is expressed as 2n, where n is a whole number. Therefore, the formula for odd numbers is expressed as, Formula for odd numbers = 2n ± 1
What is the HCF of Two Consecutive Odd Numbers?
The Highest Common Factor (HCF) of any two consecutive odd numbers is always 1. For example, the HCF of 13 and 15 is 1.
What are Even and Odd Numbers?
All whole numbers can be categorized into two parts: even and odd numbers. Even numbers are the ones whose units place digit is either 0, 2, 4, 6, or 8, and odd numbers are ones whose units place digit is either 1, 3, 5, 7, or 9. Examples of even numbers are 2, 4, 6, 8, 10, 12, 14, 16, etc, and the examples of odd numbers are 1, 3, 5, 7, 9, 11, 13, 15, 17, etc.
What is the Smallest Odd Number and the First Odd Number?
1 is the smallest odd number because the list of odd numbers starts from 1, 3, 5, 7 and so on. So, 1 is the smallest and the first odd number.
What are 3 Consecutive Odd Integers?
3 consecutive odd integers are those numbers that are odd and have a difference of 2 between them. For example 3 and 5, 11 and 13, and so on.
visual curriculum