Vertical Line
A vertical line is a line that is perpendicular to the surface or another line that is considered as the base. In coordinate geometry, the vertical lines are parallel to the y-axis and are perpendicular to the horizontal lines. A vertical line is always a straight line that goes from top to bottom or bottom to top. The vertical lines are also known as standing lines. For example, the lines joining the bases of a square or a rectangle are vertical lines.
In this article, we will explore the concept of the vertical line and its properties. We see vertical lines in our everyday life in the form of steel rails in a fence, tall trees standing upright, legs of a table, electric poles, etc. Let us learn more about it with the help of a few examples.
What is a Vertical Line?
A vertical line is a line on the coordinate plane where all the points on the line have the same x-coordinate. While plotting the points for the linear equation x = a, on a coordinate plane, we find that a vertical line is obtained on joining the coordinates. In the image below, L1 and L2 are the two vertical lines. All the points in the L1 have only 'a' as the x-coordinate (for all the values of y), and all the points in the L2 have only '-a' as the x-coordinate (for all the values of y).

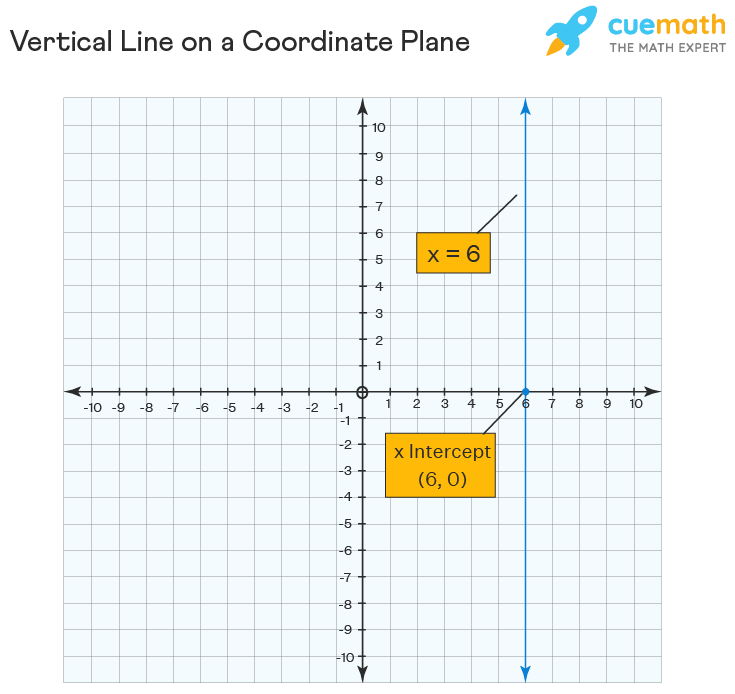
Vertical Line on a Coordinate Plane
Given the coordinates passing through a vertical line, we identify the quadrant in which the points lie. If the coordinates are denoted as (a,b), no matter whatever the value of y, the x value stays constant throughout. Thus the vertical line passes through the point 'a' on the x-axis for all values of y. The x-intercept of a vertical line x=a is the point (a,0).
Example of Vertical Lines
In the graph below, we see that for all the values of y, the x value is only 6. The vertical line is 6 units away from the origin, towards the right, and is parallel to the y-axis. (6,0,),(6,-1,),(6,-2),(6,4,),(6,8), etc... are all the points that lie on this vertical line.
Vertical Line Equation
The equation of a vertical lines is of the form "x = some number". Here, "some number" refers to the x-coordinate of any point on it. For example, the equation of a vertical line with some point (6, 2) on it is x = 6 (as shown in the above figure).
Thus, the formula for the equation of a vertical line through a point (a, b) is x = a.
Examples:
- The equation of a vertical line through (-3, 0) is x = -3.
- The equation of a vertical line through (5, -2) is x = 5.
Slope of a Vertical Line
A vertical line has a slope that is undefined. As per the definition of slope, we calculate the slope this way:
m = change in y coordinates/ change in x coordinates= (y2 - y1) / (x2 - x1)
Now, since the x-coordinate remains constant on a vertical line, therefore we have x2 = x1 = x. So, the slope of a vertical line is m = (y2 - y1) / (x - x) = (y2 - y1) / 0 which is not defined as the denominator is zero. The x coordinates remain the same for all the points on the vertical line and there is no run horizontally. Thus the slope of a vertical line is undefined.
Vertical Line Test
Vertical lines are used to find if a given graph is a function. A relation is said to be a function only when a vertical line drawn intersects the graph only at one point. A function can have only one output for every input. The image for every pre-image is unique, as per the definition of the function.
Thus if a vertical line intersects the graph at more than a point, then it is interpreted as a function having more than one output, which shows it cannot be a function. In the image below, we can witness that a vertical line drawn to the function y = f(x) is a function, because the vertical line intersects only at a point on the curve, whereas in the circle, the vertical line touches at two points. Thus the circle is not a function.
Using a vertical line to determine whether a relation is a function is termed avertical line test.

Properties of Vertical Line
- A vertical line on the coordinate plane (except the y-axis) does not have a y-intercept, because the line is parallel to the y-axis.
- The equation of vertical lines always takes the form x = a, where a is the x-intercept.
- The slope of a vertical line is undefined. Since there is no change in the x-coordinates, the denominator of the slope is zero.
- The vertical line is used to check whether a relation is a function in math.
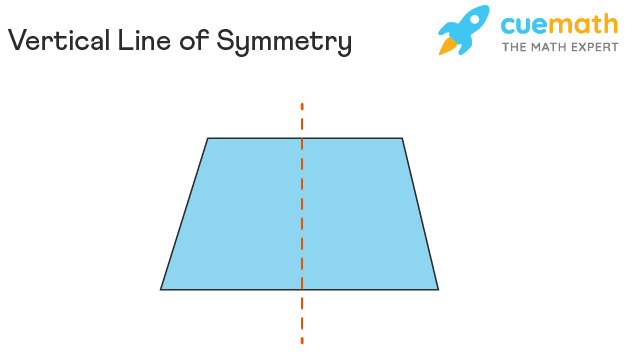
Vertical Line of Symmetry
A vertical line of symmetry is a straight line that runs top to bottom dividing the shape into two identical halves. There are 11 alphabets in English that have a vertical line of symmetry: A H I M O T U V W X Y. Below is the isosceles trapezoid which has only one vertical line of symmetry.
Important Notes on Vertical Line:
- A vertical line is a straight line perpendicular to the base.
- The equation of a vertical line is x = a, where a is the x-coordinate of any point throughout the line.
- Slope of vertical lines is not defined.
☛ Related Articles:
Examples of Vertical Line
-
Example 1. What is the equation of the vertical line passing through (-11,3)?
Solution:
The equation of the vertical line is given as x = ± a, where a is the x-intercept.
Here the x-intercept is -11.
Thus the equation of the vertical line is x = -11
We rewrite it as x + 11 = 0
Answer: The equation of the vertical line passing through (-11,3) is x + 11 = 0.
-
Example 2. Find the equation of the vertical line in the graph shown below.

Solution:
We need to identify the coordinates as represented in the graph.
The coordinates of the point are (-4,1)
Thus the equation of the vertical line is x = -4 ⇒ x + 4 = 0
Answer: The equation of the vertical line is x + 4 = 0.
-
Example 3. What is the slope of line x = -8?
Solution:
The equation of line x = -a represents a vertical line.
We know that the slope of vertical lines is undefined.
Answer: The slope of the vertical line x = -8 is undefined.

FAQs on Vertical Line
What is a Vertical Line?
A vertical line is a line on the coordinate plane where all the points on the line have the same x-coordinate, for any value of y-coordinate. Its equation is always of the form x = a where (a, b) is a point on it.
What is The Equation of a Vertical Line?
The equation of a vertical line at a point (a,0) is x = a. The equation of the vertical line is given as x = ± a, where a is the x-intercept.
What is The Slope of a Vertical Line?
The slope of a vertical line is undefined because the x-coordinates are the same. The denominator becomes zero in the calculation of slope as there is no change in x-coordinates.
What is the x-Intercept of a Vertical Line?
If the equation of a vertical line is x = a, then its x-intercept is (a, 0).
Does a Vertical Line Have a Slope?
A vertical line cannot have a slope. The slope of a vertical line is not defined.
What is The Equation of a Vertical Line Passing Through (5,2)?
The equation of a line passing through (5,2) is x = 5.
How is a Vertical Line Drawn?
A vertical line is drawn perpendicular to the baseline or to the flat surface. A vertical line goes from top to bottom or from bottom to top. On a coordinate plane, a line parallel to the y-axis is the vertical line.
What is a Vertical Line of Symmetry?
A vertical line of symmetry is a straight line that runs top to bottom dividing the shape into two identical halves.
What is the Difference Between a Vertical Line and a Horizontal Line?
Here are the differences between a vertical line and a horizontal line.
| Vertical Line | Horizontal Line |
|---|---|
| A vertical line is a line that is parallel to the y-axis. | A horizontal line is a line that is parallel to the x-axis. |
| The slope of a vertical line is not defined. | The slope of a horizontal line is 0. |
| The equation of a vertical line through a point (a, b) is x = a. | The equation of a horizontal line through a point (a, b) is y = b. |
How Do You Know The Equation of The Vertical Line Passing Through a Point?
A vertical line passes through two points having the same x-coordinates. Each point on a vertical line has the same x-coordinate. If two points have the same x-coordinate, 'a' the equation of the line is x = a. The x-intercept of a vertical line x=a is the point (a,0).
What are the Examples of Vertical Lines?
A vertical line is a line that is perpendicular to a flat surface on the ground. Flag-post, lamp-post, the standing lines in a right triangle are all a few examples of vertical lines.
What is a Vertical Line Test?
A vertical line is drawn on the graph to check if a relation is a function or not, while represented graphically. The vertical line drawn to a function on the graph intersects the curve at only one point.
visual curriculum