
Wave vector
In physics, a wave vector (also spelled wavevector) is a vector which helps describe a wave. Like any vector, it has a magnitude and direction, both of which are important: Its magnitude is either the wavenumber or angular wavenumber of the wave (inversely proportional to the wavelength), and its direction is ordinarily the direction of wave propagation (but not always, see below).
In the context of special relativity the wave vector can also be defined as a four-vector.
Definitions
Unfortunately, there are two common definitions of wave vector, which differ by a factor of 2π in their magnitudes. One definition is preferred in physics and related fields, while the other definition is preferred in crystallography and related fields. For this article, they will be called the "physics definition" and the "crystallography definition", respectively.
Physics definition
A perfect one-dimensional traveling wave follows the equation:
where:
x is position,
t is time,
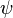 (a function of x and t) is the disturbance describing the wave (for example, for an ocean wave,
(a function of x and t) is the disturbance describing the wave (for example, for an ocean wave, 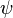 would be the excess height of the water, or for a sound wave,
would be the excess height of the water, or for a sound wave, 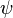 would be the excess air pressure).
would be the excess air pressure).


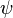 (a function of x and t) is the disturbance describing the wave (for example, for an
(a function of x and t) is the disturbance describing the wave (for example, for an 