W2

W2 or w2 can refer to:

  • Form W-2, a United States federal tax form issued by employers and stating how much an employee was paid in a year
  • Abbreviation for Wisconsin Works, a workforce development and welfare replacement program in Wisconsin (a form of Workfare; the program was adopted in the Netherlands as Work First)
  • W2, a postcode district in the W postcode area of the United Kingdom
  • Perfect World (video game), also known as PW and World2, 3D MMORPG game
  • The Vector W2, a concept car
  • British NVC community W2, a Woodland and scrub community in the British National Vegetation Classification system
  • W2 (TV), the Welsh version of television station BBC Two
  • W2, the IATA airline designator for Canadian Western Airlines
  • W2, one of four manuscripts containing the Magnus liber, or Magnus liber organi
  • w2 Concertzaal, a pop stage in 's-Hertogenbosch, The Netherlands
  • Arado W 2, a two-seat twin-engine seaplane trainer
  • Webster's New International Dictionary, Second Edition
  • Worms Reinforcements, the second game in the Worms series
    • Loading... please wait...
    This page contains text from Wikipedia, the Free Encyclopedia - https://wn.com/W2

    Parameterized complexity

    In computer science, parameterized complexity is a branch of computational complexity theory that focuses on classifying computational problems according to their inherent difficulty with respect to multiple parameters of the input or output. The complexity of a problem is then measured as a function in those parameters. This allows the classification of NP-hard problems on a finer scale than in the classical setting, where the complexity of a problem is only measured by the number of bits in the input. The first systematic work on parameterized complexity was done by Downey & Fellows (1999).

    Under the assumption that P ≠ NP, there exist many natural problems that require superpolynomial running time when complexity is measured in terms of the input size only, but that are computable in a time that is polynomial in the input size and exponential or worse in a parameter 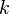k. Hence, if 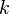k is fixed at a small value and the growth of the function over 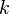k is relatively small then such problems can still be considered "tractable" despite their traditional classification as "intractable".

    Read more
    This page contains text from Wikipedia, the Free Encyclopedia - https://wn.com/Parameterized_complexity

    Podcasts:

    Email this Page Play all in Full Screen Show More Related Videos
    PLAYLIST TIME:
    ×
    ×
    ×
    ×