Breakdown

Breakdown may refer to:

Breaking down

Breakdown (vehicle), failure of a motor vehicle in such a way that it cannot be operated

Chemical decomposition, also called chemical breakdown, the breakdown of a substance into simpler components

Decomposition, the process by which tissues of a dead organism break down into simpler forms of matter

Drop set, also called a breakdown, a bodybuilding and weight training technique

Electrical breakdown, the failure of an electric circuit or a rapid reduction in the resistance of an electrical insulator that can lead to a spark

Mental breakdown, an acute, time-limited phase of exhibiting symptoms of a specific order, most commonly, depression and anxiety.

Script breakdown, a step in the production of a play, film, or comic book

Sport

Breakdown (Rugby Union), the period of open play immediately after a tackle and before and during the ensuing ruck in Rugby Union.

People

Lance Hoyt (born 1977), American professional wrestler who previously used the ring name Breakdown

This page contains text from Wikipedia, the Free Encyclopedia - https://wn.com/Breakdown

Breakdown (Seether song)

"Breakdown" is the third track and the third single from Seether's third album, Finding Beauty In Negative Spaces. Shaun Morgan has stated that the song is about his former girlfriend Amy Lee, but other sources reference a more recent relationship. To further the point, Morgan has been quoted saying "Amy and I, at our worst moments, were still better than this last girl and I at our best moments".

The song was released to radio in the middle of August 2008 and quickly became one of the most added singles to active rock radio at the time.

Music video

The music video for Breakdown premiered on November 12, 2008, on Yahoo! Music. Tony Petrossian, who directed their previous videos for "Fake It" and "Rise Above This", also made this video.

In the video, Jenna Westerbeck approaches Shaun from behind and manipulates his head, which becomes a Rubik's Cube at her touch. Throughout the video, Shaun's face changes emotions in each cube, and as the video progresses, different people (including the rest of Seether) appear in the cube, lip-syncing to the background vocals. Towards the end of the bridge, the girl opens up the cube, removing blocks featuring other women. She then finds a block that features only her, and places it in the space for Shaun's eye. She then closes the box, gives it one last spin, and the cube effect disappears. She then walks out of frame as Morgan looks at her with a sense of distaste and disappointment.

This page contains text from Wikipedia, the Free Encyclopedia - https://wn.com/Breakdown_(Seether_song)

Use Your Illusion II

Use Your Illusion II is the fourth studio album by the American rock band Guns N' Roses. It was one of two albums released in conjunction with the Use Your Illusion Tour, along with Use Your Illusion I. Bolstered by the lead single "You Could Be Mine," Use Your Illusion II was the slightly more popular of the two albums, selling 770,000 copies its first week and debuting at No. 1 on the U.S. charts, ahead of Use Your Illusion I's first week sales of 685,000. As of 2010, Use Your Illusion II has sold 5,587,000 units in the U.S., according to Nielsen SoundScan. Both albums have since been certified 7× Platinum by the RIAA. It was also No. 1 on the UK Albums Chart for a single week. It is the last Guns N' Roses album to feature rhythm guitarist Izzy Stradlin. It also included the last Guns N' Roses song to feature drummer Steven Adler, who played on "Civil War."

Overview

The Use Your Illusion albums were a stylistic turning point for Guns N' Roses (see Use Your Illusion I for discussion). In addition, Use Your Illusion II is more political than most of their previous work, with songs like "Civil War", a cover of Bob Dylan's "Knockin' on Heaven's Door", and "Get in the Ring" dealing respectively with the topics of violence, law enforcement, and media bias. The thematic material deals less with drug use than previous Guns N' Roses albums. Use Your Illusion I featured mostly songs pre-Appetite for Destruction (with notable exceptions) while Use Your Illusion II featured more tracks written during and after Appetite For Destruction.

This page contains text from Wikipedia, the Free Encyclopedia - https://wn.com/Use_Your_Illusion_II

Structure (category theory)

In mathematics, progress often consists of recognising the same structure in different contexts - so that one method exploiting it has multiple applications. In fact this is a normal way of proceeding; in the absence of recognisable structure (which might be hidden) problems tend to fall into the combinatorics classification of matters requiring special arguments.

In category theory structure is discussed implicitly - as opposed to the explicit discussion typical with the many algebraic structures. Starting with a given class of algebraic structure, such as groups, one can build the category in which the objects are groups and the morphisms are group homomorphisms: that is, of structures on one type, and mappings respecting that structure. Starting with a category C given abstractly, the challenge is to infer what structure it is on the objects that the morphisms 'preserve'.

The term structure was much used in connection with the Bourbaki group's approach. There is even a definition. Structure must definitely include topological space as well as the standard abstract algebra notions. Structure in this sense is probably commensurate with the idea of concrete category that can be presented in a definite way - the topological case means that infinitary operations will be needed. Presentation of a category (analogously to presentation of a group) can in fact be approached in a number of ways, the category structure not being (quite) an algebraic structure in its own right.

This page contains text from Wikipedia, the Free Encyclopedia - https://wn.com/Structure_(category_theory)

Algebraic structure

In mathematics, and more specifically in abstract algebra, the term algebraic structure generally refers to a set (called carrier set or underlying set) with one or more finitary operations defined on it that satisfies a list of axioms.

Examples of algebraic structures include groups, rings, fields, and lattices. More complex structures can be defined by introducing multiple operations, different underlying sets, or by altering the defining axioms. Examples of more complex algebraic structures include vector spaces, modules, and algebras.

The properties of specific algebraic structures are studied in abstract algebra. The general theory of algebraic structures has been formalized in universal algebra. Category theory is used to study the relationships between two or more classes of algebraic structures, often of different kinds. For example, Galois theory studies the connection between certain fields and groups, algebraic structures of two different kinds.

In a slight abuse of notation, the word "structure" can also refer to just the operations on a structure, instead of the underlying set itself. For example, the sentence, "We have defined a ring structure on the set 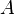$A$ ," means that we have defined ring operations on the set 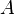$A$ . For another example, the group $(\mathbb Z, +)$ can be seen as a set $\mathbb Z$ that is equipped with an algebraic structure, namely the operation $+$ .