Torus knot
In knot theory, a torus knot is a special kind of knot that lies on the surface of an unknotted torus in R3. Similarly, a torus link is a link which lies on the surface of a torus in the same way. Each torus knot is specified by a pair of coprime integers p and q. A torus link arises if p and q are not coprime (in which case the number of components is gcd(p, q)). A torus knot is trivial (equivalent to the unknot) if and only if either p or q is equal to 1 or −1. The simplest nontrivial example is the (2,3)-torus knot, also known as the trefoil knot.
Geometrical representation
A torus knot can be rendered geometrically in multiple ways which are topologically equivalent (see Properties below) but geometrically distinct. The convention used in this article and its figures is the following.
The (p,q)-torus knot winds q times around a circle in the interior of the torus, and p times around its axis of rotational symmetry. If p and q are not relatively prime, then we have a torus link with more than one component.
Trefoil knot
In topology, a branch of mathematics, the trefoil knot is the simplest example of a nontrivial knot. The trefoil can be obtained by joining together the two loose ends of a common overhand knot, resulting in a knotted loop. As the simplest knot, the trefoil is fundamental to the study of mathematical knot theory, which has diverse applications in topology, geometry, physics, chemistry and magic.
The trefoil knot is named after the three-leaf clover (or trefoil) plant.
Descriptions
The trefoil knot can be defined as the curve obtained from the following parametric equations:
The (2,3)-torus knot is also a trefoil knot. The following parametric equations give a (2,3)-torus knot lying on torus 
:
Any continuous deformation of the curve above is also considered a trefoil knot. Specifically, any curve isotopic to a trefoil knot is also considered to be a trefoil. In addition, the mirror image of a trefoil knot is also considered to be a trefoil. In topology and knot theory, the trefoil is usually defined using a knot diagram instead of an explicit parametric equation.
Carrick mat
The carrick mat is a flat woven decorative knot which can be used as a mat or pad. Its name stems from the fact that the mat is based on the decorative-type carrick bend with the ends connected together, forming an endless knot. A larger form, called the prolong knot, is made by expanding the basic carrick mat by extending, twisting, and overlapping its outer bights, then weaving the free ends through them. This process may be repeated to produce an arbitrarily long mat.
In its basic form it is the same as a 3-lead, 4-bight Turk's head knot. The basic carrick mat, made with two passes of rope, also forms the central motif in the logo of the International Guild of Knot Tyers.
When tied to form a cylinder around the central opening, instead of lying flat, is can be used as a woggle.
See also
References
External links
Glossary of graph theory
This is a glossary of graph theory. Graph theory is the study of graphs, systems of nodes or vertices connected in pairs by edges.
!$@
A
B
C
D
E
F
G
H
I
K
κ(G) is the size of the maximum clique in G; see clique.
L
M
N
O
P
Q
R
S
T
U
V
W
References
Knot (mathematics)
In mathematics, a knot is an embedding of a circle in 3-dimensional Euclidean space, R3 (also known as E3), considered up to continuous deformations (isotopies). A crucial difference between the standard mathematical and conventional notions of a knot is that mathematical knots are closed—there are no ends to tie or untie on a mathematical knot. Physical properties such as friction and thickness also do not apply, although there are mathematical definitions of a knot that take such properties into account. The term knot is also applied to embeddings of 
in 
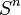, especially in the case 
. The branch of mathematics that studies knots is known as knot theory, and has many simple relations to graph theory.
Formal definition
A knot is an embedding of the circle (S1) into three-dimensional Euclidean space (R3). or the 3-sphere, S3, since the 3-sphere is compact. Two knots are defined to be equivalent if there is an ambient isotopy between them.
Projection
A knot in R3 (respectively in the 3-sphere, S3), can be projected onto a plane R2 (resp. a sphere S2). This projection is almost always regular, meaning that it is injective everywhere, except at a finite number of crossing points, which are the projections of only two points of the knot, and these points are not collinear. In this case, by choosing a projection side, one can completely encode the isotopy class of the knot by its regular projection by recording a simple over/under information at these crossings. In graph theory terms, a regular projection of a knot, or knot diagram is thus a 4-valent planar graph with over/under decorated vertices. The local modifications of this graph which allow to go from one diagram to any other diagram of the same knot (up to ambient isotopy of the plane) are called Reidemeister moves.
Heraldic knot
A heraldic knot (referred to in heraldry as simply a knot) is a knot, unknot, or design incorporating a knot used in European heraldry. While a given knot can be used on more than one family's achievement of arms, the family on whose coat the knot originated usually gives its name to the said knot (the exception being the Tristram knot). These knots can be used to charge shields and crests, but can also be used in badges or as standalone symbols of the families for whom they are named (like Scottish plaids). The simplest of these patterns, the Bowen knot, is often referred to as the heraldic knot in symbolism and art outside of heraldry.
Podcasts:
