
The Kingdom of Solomon
The Kingdom of Solomon is an Iranian religious/historical film trilogy, produced by Mojtaba Faravardeh and directed by Shahriar Bahrani who has made Saint Mary before. The Kingdom of Solomon was going to be released internationally on November 2010 after its screening in Iran, but due to some technicalities its global release has been delayed. The film tells the life story of Prophet Solomon, the King of Israelites. It is mostly based on the Islamic accounts of Solomon's prophetic life extracted from the Qur'an but it also draws upon parallels found in some Jewish texts.
Plot
Solomon is a wise prophet selected as the crown prince by his father King David (Dawud in Islamic texts) when he was 9. Following Prophet David's death, Solomon succeeds to the crown and God appoints him as a prophet. Requesting from God the establishment of a divine kingdom, Solomon takes the wind under his command and jinns and demons under his control. Inviting rulers of the neighbouring lands to the monotheistic religion, Prophet Solomon continues his divine mission in as much as Balqis, the Queen of Sheba professes monotheism. At the end, while leaning on his cane, Solomon bids farewell to the world, and the jinns and demons get out of reign and return to their own world.
Solomon (Karaite prince)
Solomon ben David (Hebrew: שלמה בן דוד) was a Karaite leader of the late tenth and early eleventh centuries CE. He was the son of David ben Boaz. As a direct lineal descendant of Anan ben David, he was regarded as nasi and resh galuta of the Karaite community. He was succeeded by his son Hezekiah ben Solomon.
Solomon (disambiguation)
Solomon is a figure identified in the Hebrew Bible, Baha'i scripture and in the Quran as a king of Israel, and the son of David.
Solomon may also refer to:
People
Places
Other
Glossary of graph theory
This is a glossary of graph theory. Graph theory is the study of graphs, systems of nodes or vertices connected in pairs by edges.
!$@
A
B
C
D
E
F
G
H
I
K
κ(G) is the size of the maximum clique in G; see clique.
L
M
N
O
P
Q
R
S
T
U
V
W
References

Knot (mathematics)
In mathematics, a knot is an embedding of a circle in 3-dimensional Euclidean space, R3 (also known as E3), considered up to continuous deformations (isotopies). A crucial difference between the standard mathematical and conventional notions of a knot is that mathematical knots are closed—there are no ends to tie or untie on a mathematical knot. Physical properties such as friction and thickness also do not apply, although there are mathematical definitions of a knot that take such properties into account. The term knot is also applied to embeddings of  in
in 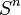 , especially in the case
, especially in the case  . The branch of mathematics that studies knots is known as knot theory, and has many simple relations to graph theory.
. The branch of mathematics that studies knots is known as knot theory, and has many simple relations to graph theory.
Formal definition
A knot is an embedding of the circle (S1) into three-dimensional Euclidean space (R3). or the 3-sphere, S3, since the 3-sphere is compact. Two knots are defined to be equivalent if there is an ambient isotopy between them.
Projection
A knot in R3 (respectively in the 3-sphere, S3), can be projected onto a plane R2 (resp. a sphere S2). This projection is almost always regular, meaning that it is injective everywhere, except at a finite number of crossing points, which are the projections of only two points of the knot, and these points are not collinear. In this case, by choosing a projection side, one can completely encode the isotopy class of the knot by its regular projection by recording a simple over/under information at these crossings. In graph theory terms, a regular projection of a knot, or knot diagram is thus a 4-valent planar graph with over/under decorated vertices. The local modifications of this graph which allow to go from one diagram to any other diagram of the same knot (up to ambient isotopy of the plane) are called Reidemeister moves.

Heraldic knot
A heraldic knot (referred to in heraldry as simply a knot) is a knot, unknot, or design incorporating a knot used in European heraldry. While a given knot can be used on more than one family's achievement of arms, the family on whose coat the knot originated usually gives its name to the said knot (the exception being the Tristram knot). These knots can be used to charge shields and crests, but can also be used in badges or as standalone symbols of the families for whom they are named (like Scottish plaids). The simplest of these patterns, the Bowen knot, is often referred to as the heraldic knot in symbolism and art outside of heraldry.
Podcasts:
Solomon
ALBUMS
- Chillout on Reggae released: 2008
Solomon
ALBUMS
- The Wrestler released: 2009
- Down at the Twisted AM Lounge released: 2007
- Homegrown: Volume 1: Mixed by Skitz released: 2004
- Countryman released: 2001
- Schöner Hören 08 released:
- We Don't Know What's Coming released:
Homegrown: Volume 1: Mixed by Skitz
Released 2004- Intro
- Dogz n Sledgez
- Muzik Ed Special
- Soldiers
- Bakardi Slang
- Revolution
- Norse Code
- Sionara (Skitz Xclusive mix)
- Just Me
- You Done Know (Live-O)
- The Greatest Show
- Bubble
- Agent Orange
- Check It
- Champion Sound
- Warrior (dirty version)
- So U Want More? (Revox)
- Slumber (Skitz Xclusive mix)
- Big Black Boots
- What's Goin On
- Introducing Mr Tibbs
- What's My Life Like
Solomon
ALBUMS
- Christian Deathcore: Volume 1 released: 2012
Christian Deathcore: Volume 1
Released 2012- Conquer
- Like Father, Like Son
- Destroyer
- The Blood Has Been Shed
- Cell Games
- The East and the West
- A Will to Find
- Defamation
- Rift
- Author
- Laminin
- Counter
- You're Not a Popstar
- Zodiac
- Acquit
- Missing Rungs
- The War of Dominance
- Redeemed
- The Brutal Truth
- Abomination Recreation
- Anyone Unnecessary Fate
- The Bay Harbor Butcher
- Buried as Saints
- Welcome to Hell
- Darkness/Reversal
- City of False Prophets
- Ministers of Sedition
- Confusion
- Letters
- Junior (The Fallen)
- Exhortacion
- Change Your Life (Or Change Your Attitude)
- Sifonia do silencio
- Unity
- Tiempo de cambiar
- Martyrdom
- To Be Sold Out
- False Prophet
- Revival
- Slumber (midi version)
Solomon
ALBUMS
- Die Berühmten Klavierwerke released: 2000
- Piano Concertos Nos. 2-5 / Piano Sonata No. 14 released: 1995
- The Complete Recordings of Chopin released: 1994
- Piano Sonatas opp. 90, 101, 106, 109, 110, 111 released: 1993
- Piano Concertos released: 1991
- Great Pianists of the 20th Century, Volume 92: Solomon released:
- Piano Sonatas Nos. 26, 27 & 29 released:
Die Berühmten Klavierwerke
Released 2000- Sonate für Klavier Nr.1 C-Dur Op. 1: I. Allegro
- Sonate für Klavier Nr.1 C-Dur Op. 1: II. Andante
- Sonate für Klavier Nr.1 C-Dur Op. 1: III. Scherzo
- Sonate für Klavier Nr.1 C-Dur Op. 1: IV. Finale
- Sonate für Klavier Nr.2 fis-Moll Op. 2: I. Allegro non troppo, ma energico
- Sonate für Klavier Nr.2 fis-Moll Op. 2: II. Andante con espressione
- Sonate für Klavier Nr.2 fis-Moll Op. 2: III. Scherzo
- Sonate für Klavier Nr.2 fis-Moll Op. 2: IV. Finale
- Variationen über ein eigenes Thema Nr.1 D-Dur Op. 21/1
Piano Concertos Nos. 2-5 / Piano Sonata No. 14
Released 1995- Piano Concerto No. 3 in C minor, Op. 37: I. Allegro con brio
- Piano Concerto No. 3 in C minor, Op. 37: II. Largo
- Piano Concerto No. 3 in C minor, Op. 37: III. Rondo. Allegro
- Piano Concerto No. 5 in E-flat major, Op. 73 "Emperor": I. Allegro
- Piano Concerto No. 5 in E-flat major, Op. 73 "Emperor": II. Adagio un poco mosso
- Piano Concerto No. 5 in E-flat major, Op. 73 "Emperor": III. Rondo. Allegro
The Complete Recordings of Chopin
Released 1994- Études, Op. 10-No. 3 in E major
- Études, Op. 10-No. 8 in F major
- Études, Op. 10-No. 9 in F minor
- Études, Op. 25-No. 1 in A flat major
- Études, Op. 25-No. 2 in F minor
- Études, Op. 25-No. 3 in F major
- Études, Op. 25-No. 3 in F major
- Nocturne in E flat major, Op. 9 No. 2
- Nocturne in D flat major, Op. 27 No. 2
- Valse in A flat major, Op. 42
- Valse in E minor, Op. posth.
- Polonaise in A major, Op. 40 No. 1
- Polonaise in A flat major, Op. 53
- Fantaisie [sic] in F minor, Op. 49
- Mazurka in A minor, Op. 68 No. 2
- Ballade No. 4 in F minor, Op. 52
- Berceuse, Op. 57
Piano Sonatas opp. 90, 101, 106, 109, 110, 111
Released 1993- Piano Sonata No. 28 in A major, op. 101: I. Allegretto, ma non troppo
- Piano Sonata No. 28 in A major, op. 101: II. Vivace alla Marcia
- Piano Sonata No. 28 in A major, op. 101: III. Adagio, ma non troppo, con affetto
- Piano Sonata No. 28 in A major, op. 101: IV. Allegro
- Piano Sonata No. 29 in B-flat major, op. 106: I. Allegro
- Piano Sonata No. 29 in B-flat major, op. 106: II. Scherzo. Assai vivace-Presto
- Piano Sonata No. 29 in B-flat major, op. 106: III. Adagio sostenuto
- Piano Sonata No. 29 in B-flat major, op. 106: IV. Largo-Allegro-Allegro risoluto
Piano Concertos
Released 1991- Piano Concerto in B-flat major, op. 58: I. Allegro con brio
- Piano Concerto in B-flat major, op. 58: II. Adagietto
- Piano Concerto in B-flat major, op. 58: III. Andante maestoso - Molto vivo
- Piano Concerto in F-sharp minor, op. 20: I. Allegro
- Piano Concerto in F-sharp minor, op. 20: II. Theme: Andante - Variation I: Allegro scherzando - Variation II: Allegro scherzando / Variation III: Adagio / Variation IV: Allegretto - Tempo I
- Piano Concerto in F-sharp minor, op. 20: III. Allegro moderato
- Hungarian Fantasy, S. 123
Great Pianists of the 20th Century, Volume 92: Solomon
- Prelude and Fugue in A minor, BWV 543: Prelude
- Prelude and Fugue in A minor, BWV 543: Fugue
- Sonata No. 13 in B-flat major, K. 333: I. Allegro
- Sonata No. 13 in B-flat major, K. 333: II. Andante cantabile
- Sonata No. 13 in B-flat major, K. 333: III. Allegretto grazioso
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": I. Allegro
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": II. Scherzo. Assai vivace
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": III. Adagio sostenuto
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": IV. Largo
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": V. Allegro risoluto
Piano Sonatas Nos. 26, 27 & 29
- Sonata No. 26 in E-flat major, Op. 81a "Les Adieux": I. Adagio – Allegro "Les Adieux"
- Sonata No. 26 in E-flat major, Op. 81a "Les Adieux": II. Andante espressivo "L'Absence"
- Sonata No. 26 in E-flat major, Op. 81a "Les Adieux": III. Vivacissimamente – Poco Andante "Le Retour"
- Sonata No. 27 in E minor, Op. 90: I. Con vivacita, ma sempre con sentimento ed espressione
- Sonata No. 27 in E minor, Op. 90: II. Non tanto mosso e molto cantabile
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": I. Allegro
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": II. Scherzo
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": III. Adagio e sostenuto
- Sonata No. 29 in B-flat major, Op. 106 "Hammerklavier": IV. Largo – Un poco piu vivace – Allegro – Tempo – Prestissimo – Allegroris
Born: 1902-08-06
Died: 1988-02-22

