Snub icosidodecadodecahedron

In geometry, the snub icosidodecadodecahedron is a nonconvex uniform polyhedron, indexed as U₄₆.

Cartesian coordinates

Cartesian coordinates for the vertices of a snub icosidodecadodecahedron are all the even permutations of

with an even number of plus signs, where

and where τ = (1+√5)/2 is the golden mean and ρ is the real solution to ρ³=ρ+1, or approximately 1.3247180. ρ is called the plastic constant. Taking the odd permutations of the above coordinates with an odd number of plus signs gives another form, the enantiomorph of the other one.

Related polyhedral

Medial hexagonal hexecontahedron

The medial hexagonal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the uniform snub icosidodecadodecahedron.

See also

References

External links

Four-sided die

Four-sided dice, abbreviated d4, are often used in tabletop role-playing games to obtain random integers in the range 1–4. Two forms exist of this die: a tetrahedron (pyramid shape) with four equilateral triangle-shaped faces, and an elongated long die with four faces. The former type does not roll well and is thus usually thrown into the air or shaken in a box.

Historical

Four-sided dice were among the gambling and divination tools used by early man who carved them from nuts, wood, stone, ivory and bone. Six-sided dice were invented later but four-sided dice continued to be popular in Asia. In Ancient Rome, elongated four-sided dice were called tali while the six-sided cubic dice were tesserae. In India and Tibet, three four-sided long dice were rolled sequentially as an oracle, to produce 1 of 64 possible outcomes. The ancient Jewish dreidel is a four-sided long die with one end changed into a handle, to allow it to be spun like a top.

The ancient Egyptian Royal Game of Ur uses eight four-sided pyramid-shaped dice made out of rock, half of them colored white, and half black. The Scandinavian game daldøs uses a four-sided long die.

2-sided

In topology, a compact codimension one submanifold of a manifold is said to be 2-sided in when there is an embedding

with 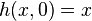for each 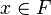and

In other words, if its normal bundle is trivial.

This means, for example that a curve in a surface is 2-sided if it has a tubular neighborhood which is a cartesian product of the curve times an interval.

A submanifold which is not 2-sided is called 1-sided.

Examples

Surfaces

For curves on surfaces, a curve is 2-sided if and only if it preserves orientation, and 1-sided if and only if it reverses orientation: a tubular neighborhood is then a Möbius strip. This can be determined from the class of the curve in the fundamental group of the surface and the orientation character on the fundamental group, which identifies which curves reverse orientation.