For the optimization algorithm, see Gradient descent.

In mathematics, the method of steepest descent or stationary phase method or saddle-point method is an extension of Laplace's method for approximating an integral, where one deforms a contour integral in the complex plane to pass near a stationary point (saddle point), in roughly the direction of steepest descent or stationary phase. The saddle-point approximation is used with integrals in the complex plane, whereas Laplace’s method is used with real integrals.

The integral to be estimated is often of the form

Failed to parse (Missing texvc executable; please see math/README to configure.): \displaystyle \int_Cf(z)e^{\lambda g(z)}dz

where C is a contour and λ is large. One version of the method of steepest descent deforms the contour of integration so that it passes through a zero of the derivative g′(z) in such a way that on the contour g is (approximately) real and has a maximum at the zero.

The method of steepest descent was first published by Debye (1909), who used it to estimate Bessel functions and pointed out that it occurred in the unpublished note Riemann (1863) about hypergeometric functions. The contour of steepest descent has a minimax property, see Fedoryuk (2001). Siegel (1932) described some other unpublished notes of Riemann, where he used this method to derive the Riemann-Siegel formula.

Extensions and generalizations [link]

An extension of the steepest descent method is the so-called nonlinear stationary phase/steepest descent method. Here, instead of integrals, one needs to evaluate asymptotically solutions of Riemann–Hilbert factorization problems.

Given a contour C in the complex sphere, a function ƒ defined on that contour and a special point, say infinity, one seeks a function M holomorphic away from the contour C, with prescribed jump across C, and with a given normalization at infinity. If ƒ and hence M are matrices rather than scalars this is a problem that in general does not admit an explicit solution.

An asymptotic evaluation is then possible along the lines of the linear stationary phase/steepest descent method. The idea is to reduce asymptotically the solution of the given Riemann–Hilbert problem to that of a simpler, explicitly solvable, Riemann–Hilbert problem. Cauchy's theorem is used to justify deformations of the jump contour.

The nonlinear stationary phase was introduced by Deift and Zhou in 1993, based on earlier work of the Russian mathematician Alexander Its. A (properly speaking) nonlinear steepest descent method was introduced by Kamvissis, K. McLaughlin and P. Miller in 2003, based on previous work of Lax, Levermore, Deift, Venakides and Zhou. As in the linear case, steepest descent contours solve a min-max problem.

The nonlinear stationary phase/steepest descent method has applications to the theory of soliton equations and integrable models, random matrices and combinatorics.

References [link]

Debye, P (1909), "Näherungsformeln für die Zylinderfunktionen für große Werte des Arguments und unbeschränkt veränderliche Werte des Index", Mathematische Annalen 67 (4): 535–558, DOI:10.1007/BF01450097 English translation in Debye, Peter J. W. (1954), The collected papers of Peter J. W. Debye, Interscience Publishers, Inc., New York, ISBN 978-0-918024-58-9, MR 0063975
Deift, P.; Zhou, X. (1993), "A steepest descent method for oscillatory Riemann-Hilbert problems. Asymptotics for the MKdV equation", Ann. Of Math. (The Annals of Mathematics, Vol. 137, No. 2) 137 (2): 295–368, DOI:10.2307/2946540, JSTOR 2946540 .
Erdelyi, A. (1956), Asymptotic Expansions, Dover .
Fedoryuk, M.V. (2001), "Steepest_descent,_method_of&oldid=13529", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4, https://www.encyclopediaofmath.org/index.php?title=Steepest_descent,_method_of&oldid=13529
Kamvissis, S.; McLaughlin, K. T.-R.; Miller, P. (2003), "Semiclassical Soliton Ensembles for the Focusing Nonlinear Schrödinger Equation", Annals of Mathematics Studies (Princeton University Press) 154 .
Riemann, B. (1863), Sullo svolgimento del quoziente di due serie ipergeometriche in frazione continua infinita (Unpublished note, reproduced in Riemann's collected papers.)
Siegel, C. L. (1932), "Über Riemanns Nachlaß zur analytischen Zahlentheorie", Quellen Studien zur Geschichte der Math. Astron. und Phys. Abt. B: Studien 2: 45–80 Reprinted in Gesammelte Abhandlungen, Vol. 1. Berlin: Springer-Verlag, 1966.

https://wn.com/Method_of_steepest_descent

Saddle point

In mathematics, a saddle point is a point in the domain of a function that is a stationary point but not a local extremum. The name derives from the fact that the prototypical example in two dimensions is a surface that curves up in one direction, and curves down in a different direction, resembling a saddle or a mountain pass. In terms of contour lines, a saddle point in two dimensions gives rise to a contour that appears to intersect itself.

Mathematical discussion

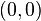A simple criterion for checking if a given stationary point of a real-valued function F(x,y) of two real variables is a saddle point is to compute the function's Hessian matrix at that point: if the Hessian is indefinite, then that point is a saddle point. For example, the Hessian matrix of the function $z=x^2-y^2$ at the stationary point $(0, 0)$ is the matrix

which is indefinite. Therefore, this point is a saddle point. This criterion gives only a sufficient condition. For example, the point 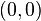$(0, 0)$ is a saddle point for the function 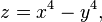$z=x^4-y^4,$ but the Hessian matrix of this function at the origin is the null matrix, which is not indefinite.

This page contains text from Wikipedia, the Free Encyclopedia - https://wn.com/Saddle_point

location on Google Map

Saddle Point

Saddle Point (53°1′S 73°29′E / 53.017°S 73.483°E / -53.017; 73.483Coordinates: 53°1′S 73°29′E / 53.017°S 73.483°E / -53.017; 73.483) is a rocky point separating Corinthian Bay and Mechanics Bay on the north coast of Heard Island in the Antarctic.

The terminus of Challenger Glacier is located at the eastern side of Corinthian Bay, close west to Saddle Point. To the east of Challenger Glacier is Downes Glacier, whose terminus is located at Mechanics Bay, between Saddle Point and Cape Bidlingmaier.