Partition of a set

In mathematics, a partition of a set is a grouping of the set's elements into non-empty subsets, in such a way that every element is included in one and only one of the subsets.

Definition

A partition of a set X is a set of nonempty subsets of X such that every element x in X is in exactly one of these subsets (i.e., X is a disjoint union of the subsets).

Equivalently, a family of sets P is a partition of X if and only if all of the following conditions hold:

In mathematical notation, these conditions can be represented as

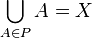
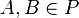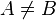if and then ,

where 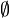is the empty set.

The sets in P are called the blocks, parts or cells of the partition.

The rank of P is |X| − |P|, if X is finite.

Examples