Given in Fig. 1 is a radar block diagram that captures the essential components affecting Z_DR. The vertical dashed lines mark measurement planes that are useful for defining the various gains and powers of the radar.

where TX_H,V are the transmit powers measured at plane 2, GH,VA are the H and V antenna gains, and RX_H,V are the H and V receiver gains from plane 2 to plane 1. The differential gains are defined as

so that the Z_DR bias (also referred to as the Z_DR offset) can be written as

in linear form, and in dB as

The Z_DR offset is determined using a birdbath scan. The key assumption of the method is that Z_DR is 0 when looking vertically at falling precipitation. A deviation from 0 dB is then attributed to a Z_DR offset or bias. The differential bias in the Δ_RX path can be due to differential receiver gain, circulator attenuation, and LNAs and other electronics in H and V electronic paths. Offset in Δ_TX is primarily a function of the power divider circuitry and the circulators. All of these components have temperature-dependent gains. The differential offset due to Δ_A is due to uncertainties in the antenna characterization (H and V antenna gain, width of the main lobe) and antenna temperature (we will discuss this later).

Z_DR bias estimated from a birdbath scan is computed as a range-averaged Z_DR in the antenna far field (starting at about a 700 m range). Threshold requirements are ρ_hv>0.9 (copolar correlation coefficient; Bringi and Chandrasekar, 2001), SQI >0.5 (signal quality index or normalized coherent power¹) and at least 10 valid range bins (25 m length each) in a ray. Then the median Z_DR is computed from all rays of the sweep. In order to obtain the diurnal-averaged Z_DR, the median Z_DR from all birdbath scans of a given day is computed. A median Z_DR is computed only if there are at least six birdbath scans available with valid data. There is no further separation according to the hydrometeors as the method is also applicable to mixed-phase and solid-phase precipitation (e.g., Dixon et al., 2018).

The automated procedure to adjust the Z_DR offset on a diurnal bias has been implemented. If the offset Δ_TX,RX is properly set initially on a given day, we have

where Δ_TX,RX is the static offset which is stored as an initial parameter in the signal processor and ZDR90∘,ti is the current diurnal Z_DR value determined from the birdbath scan. Δ_TX,RX is a value that has been determined during routine maintenance.

If the following is found at a given day t_i,

then the offset needs to be adjusted. The nonzero Z_DR offset is now

In order to calibrate Z_DR, the initial Z_DR offset is corrected. The new system offset ΔTX,RX,ti is then

The correct Z_DR offset is stored in the metadata set of every ODIM HDF5 sweep that is sent to the central DWD radar data processing site. As part of centralized quality control, this offset is applied to the Z_DR data prior to any product generation. The corrected offset is valid until a new Z_DR offset can be computed from birdbath data. Depending on the season, weeks may pass until the next precipitation event that can be used to assess the Z_DR offset occurs. In principle there could be drifts in the Z_DR bias which would introduce a Z_DR bias during a dry period. However our experience indicates that the radar hardware state is sufficiently stable and precipitation is frequent enough so that the mean Z_DR bias over a day would be smaller than 0.1 dB in these dry periods. This is further discussed in Sect. 8.

In this section the configuration and setup of the solar box scans is described. The scanning azimuth and elevation limits of a box scan are 8^∘ and 5^∘, respectively, centered on the position of the sun at the beginning of the scan. The scan takes about 4 min to complete and is scheduled every 10 min. During this time the scan box azimuth and elevation limits are not adjusted to account for the movement of the sun; however, in the postcollection analysis of the data, the movement of the sun is corrected by using the ray time tag from which the position of the sun is determined. The radar operates with a pulse length of 0.8 µs (0.4 µs), with a scan rate of 2^∘ s⁻¹ (0.1^∘ s⁻¹) and a PRF (pulse repetition frequency) of 800 Hz (1600 Hz). The elevation angle increment is 0.1^∘. The range resolution is set to 250 m, and data up to a range of 150 km are acquired.

The system is transmitting while scanning, and the PRF is chosen such that the system is operated with a constant duty cycle. It is important that the transmitter operates while making solar scans so that the radar components of the transmit and receiver paths are in an operational state.

The integrated solar powers are corrected for noise. Prior to each solar scan, the thermal background noise is estimated at the elevation of the sun and about 30^∘ off the azimuth of the sun. Overcast situations do not bias the solar scans. In the analysis the signal-to-noise ratios SNRh and SNRv (horizontal and vertical, respectively), the cross-correlation coefficient ρ_hv, and the differential solar power S (stored as Z_DR) are used. All data moments are computed from unfiltered time series (no Doppler clutter filter is applied). To avoid ground clutter contamination, only data beyond a 50 km range are used; however, at low-elevation angles data beyond a 50 km range can be contaminated by clutter. If this is the case, those data are removed from the analysis.

The standard lightning protection of a radar system consists of four vertical lightning poles in the vicinity of the radome which extend above the highest point of the radome. For the Hohenpeißenberg radar system, prior the measurements used in this study, three of the four lightning poles were removed in order to avoid disturbance of the radar data due to the lightning protection hardware.

The typical solar beam plots for the SNRh and SNRv patterns are shown in Fig. 2. The corresponding differential solar power S pattern and the cross-correlation coefficient ρ_hv are shown in Fig. 3.

Figure 2Typical SNR solar beam plot from a box scan, showing SNRh (a) and SNRv (b). These plots illustrate how the solar beamwidths are computed. On top of each panel, the corresponding position of the sun relative to the radar, the computed elevation (el) and azimuth (az) radar positioning bias, the transmit frequency, the radome temperature, and the Z_DR integrated over 0.5 and 1^∘ radii is given as a standard information data set with this product. The red and blue crosses indicate the azimuth and elevation bias for the two polarizations.

Download

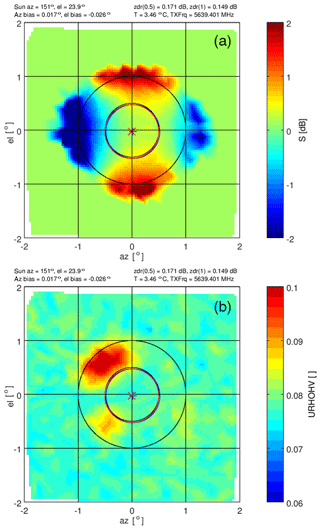
Figure 3The corresponding pattern of differential solar power S is shown in panel (a) (to be compared with Fig. 2). The cross-channel correlation coefficient ρhvS is shown in panel (b) (denoted as URHOHV on the color scale label). Note the narrow scale from 0 to 0.1.

Download

There are four areas of large differential powers at a radii of 1^∘ (Fig. 3). This differential solar power S pattern is supported by analyzing the 3 dB beamwidth employing the method of Huuskonen et al. (2014). The time series of 3 dB beamwidths from 91 solar box scans are shown in Fig. 4, and the corresponding azimuth and elevation of the sun during that day are shown in Fig. 5. We show the series in order to illustrate the consistency of the results throughout a day for different elevations and azimuths. The mean azimuth width is 0.94^∘ ± 0.01^∘ and 0.98^∘ ± 0.01^∘ for H and V, respectively. The elevation width in H and V is 0.95^∘ ± 0.006^∘ and 0.89^∘ ± 0.006^∘, respectively.

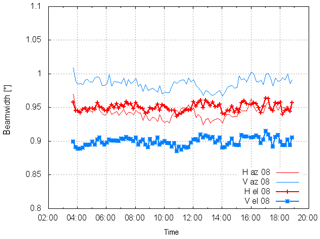
Figure 4Time series (UTC) of 3 dB beamwidths from 91 solar box scans. Data are taken on 23 June 2018. Shown are the beamwidths in vertical and horizontal dimensions and for horizontal and vertical polarizations.

Download

Figure 5The diurnal cycle (time in UTC) of the elevation and azimuth positions of the sun (23 June 2018) which correspond to the solar box scans that are used to compute the beamwidths in Fig. 4.

Download

The beamwidth results indicate a near-circular beam shape in H, and in contrast, the beam shape is more elliptical in V. The superposition of the circular and elliptical beam shapes leads to the observed solar Z_DR or S pattern.

During the Hohenpeißenberg acceptance tests a series of antenna pattern measurements were carried out (Frech et al., 2013). An example S pattern is shown in Fig. 6. The dynamic range of an antenna pattern measurement is of course much larger (peak SNR of the external source is 68 dB compared to the peak solar SNR of about 7 dB), so there is differential power visible outside the main beam. But within the main beam (±1^∘) the S patterns show a remarkable agreement. Thus, the main lobe of solar S patterns can be used to assess the antenna performance without carrying out dedicated antenna pattern measurements. Taking such a measurement on a regular basis throughout the lifetime of a radar system (≈20 years) helps to monitor the state of the antenna assembly.

Figure 6Hohenpeißenberg Z_DR antenna pattern (UZDR) taken on 18 May 2011, to be compared with the pattern of differential solar power (Fig. 3a). See also Fig. 2.

Download

The complex H and V time-series data resulting from scanning the solar disk can also be used to create a cross-channel correlation antenna pattern. The simultaneously received voltage time series from a single dwell angle, V_h(i) and V_v(i), for the horizontal and vertical channels, respectively, are correlated as

where N is the number of samples. Thus ρhvS gives the pointwise (spatial) correlation from temporal averages. These correlation data from all dwell angles are interpolated to a grid. The resulting magnitude of the correlation product of Eq. (21) is shown in Fig. 3b. If solar radiation is unpolarized, the correlation of data between any two orthogonal receive polarization channels is zero by definition. The correlation magnitude in Fig. 3b shows two principal lobes in the two left quadrants where the correlation increases. These two areas of increased correlation coefficient are manifestations of the antenna polarization errors (Hubbert et al., 2010a, b). This signature is present throughout nearly all of the solar box scan measurements.

Over 2157 solar box scans were made in order to study the variability in S. Before a statistical analysis is performed on these data, two illustrative S time series from 2 particular days are shown. The example from 3 June 2018 is shown in Fig. 7. The mean differential solar power S is −0.19 dB, and over the day the standard deviation is 0.032 dB. The standard deviation was determined after the removal of the trend using a 5th order polynomial. In addition two temperature measurements are shown. One is termed the radome temperature which indicates the temperature in the radome to which the antenna assembly is exposed. The LNA (low-noise amplifier) temperature is a temperature reading close to the LNAs within the receiver box which is assumed to be representative for the temperature condition within the receiver box. By eye there seems to be a correlation between temperature and S. From similar data gathered on 15 June 2018 in Fig. 8, this correlation appears more obvious. S increases by about 0.2 dB over this day with a temperature increase of about 7 ^∘C. For this case the mean S is −0.15 dB and the standard deviation is 0.038 dB. In principle we would expect a constant S throughout the day because the sun is an unpolarized source of radiation. So besides the obvious temperature dependence, the variability in S (expressed in terms of the standard deviation) may be caused by the radome, insufficient cross-polar isolation of the antenna and/or clutter, or just random sampling errors. There are no means of separating those effects with the existing measurements.

Figure 7Variability in differential solar power S (dB) on 3 June 2018 (UTC). Also shown is the radome temperature and the temperature near the LNA in the receiver box.

Download

Figure 8Variability in differential solar power S [dB] on 15 June 2018 (UTC). Also shown is the radome temperature and the temperature near the LNA in the receiver box.

Download

Next, the time series of S² are compared to the operational monitoring results of differential solar power S², which are based on solar interferences identified from the operational scanning data, and to Z_DR data from birdbath scans (S is squared to make it comparable to Z_DR). In order to compare S from box scans to the operational results, the system Z_DR offset of −0.1 dB is subtracted from those data. There is only one S² and Z_DR value per day from operational monitoring, indicated by the two straight lines in Figs. 9 and 10, respectively. Z_DR from the birdbath scans is only updated if there has been sufficient precipitation on the previous day. Otherwise the Z_DR of the last precipitation event is assumed to be still valid.

There is on average a very good agreement between the S² derived from operational scanning and the S² from the box scans (Figs. 9 and 10). It is obvious that diurnal temperature variations cannot be captured by the S² which is derived from operational scanning. The respective Z_DR from the birdbath scan on 3 June is near 0 dB compared to −0.1 dB for S² . The opposite is found on 15 June, when the birdbath Z_DR is −0.1 dB and S² is near 0 dB.

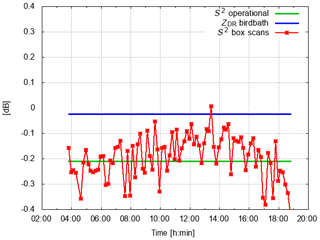
Figure 9S² variability during 3 June 2018 (UTC) compared to the S² and Z_DR from operational monitoring. Operational data are updated once a day. No update of Z_DR is possible if there has not been sufficient precipitation over the radar site on the previous day. In that case we keep the Z_DR from the last precipitation event (here 1 June 2018).

Download

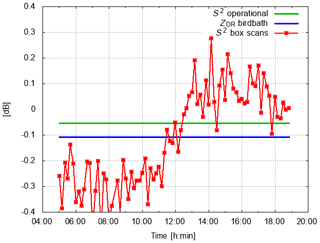
Figure 10S² variability during 15 June 2018 (UTC) compared to the S² and Z_DR derived from operational monitoring. Operational data are updated once a day. No update of Z_DR is possible if there has not been sufficient precipitation over the radar site on the previous day. In that case we keep the Z_DR from the last precipitation event (here 13 June 2018).

Download

The antenna gain is computed using the measured solar power by the radar and the DRAO solar flux (see Eq. 20). If the receive path is properly characterized and calibrated, the retrieved antenna gain should be the antenna gain as provided by the antenna manufacturer. If the solar power based on the DRAO data is used to compute the antenna gain using Eq. (20), a deviation from the gain value provided by the manufacturer is due to the assumptions made about the antenna characteristics. Similarly, by using the radar-measured solar power, a systematic deviation from the manufacturer gain is indicative of a calibration error.

The antenna gain results are shown in Figs. 11 and 12 for the same dates as before, where the time series of gain for both polarizations are shown as a function of the solar azimuth position. The antenna gain results (about 90 estimates based on 90 box scans) in Figs. 11 and 12 are compared to the gain estimate using the solar power derived from the operational monitoring and the gain estimate based on DRAO solar power data, for which there is just one value per day, indicated by the straight lines in Figs. 11 and 12. For both the 3 June and 15 June 2018 data, larger variability in gain on the order of 0.2 dB is observed in the morning and evening when the elevation of the sun is low. Most likely surface clutter is the prime contribution to this variation in gain. A decrease in gain on the order of 0.3 dB is observed during the day, followed by an increase in gain of about 0.3 dB in the evening hours.

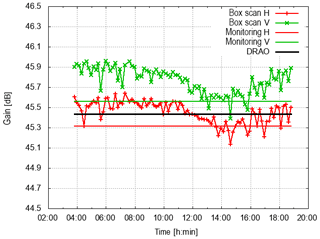
Figure 11Antenna gain time series in H and V during 3 June 2018 (UTC). Also shown is the antenna gain estimate from operational monitoring (monitoring H, monitoring V) and the gain estimate using DRAO data only.

Download

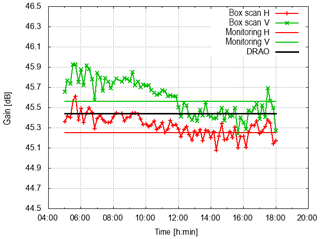
Figure 12Antenna gain time series in H and V during 15 June 2018 (UTC). Also shown is the antenna gain estimate from operational monitoring (monitoring H, monitoring V) and the gain estimate using DRAO data only.

Download

The antenna gain provided by the manufacturer is 45.4 dB in H and 45.2 dB in V. The average gain based on the box scan data in comparison to the gain based on the operational monitoring of solar hits is summarized in Table 1. Based on those measurements, there is a very good agreement between the gain estimates of H using solar power measured by radar and the manufacturer value of 45.4 dB.

Therefore, the assumptions about the antenna characteristics appear reasonable. The deviation is within 0.2 dB (considering the gain in V). The gain estimate from the solar box scans is about 0.3–0.4 dB larger in V than the estimate in H and the manufacturer gain. This suggests a receiver calibration bias on this order which indicates that the receive channel is actually more sensitive (meaning that dBm0 should be smaller by 0.3–0.4 dB).

Qualitatively, the diurnal variation in gain seems to suggest that there is a temperature correlation with a decrease in gain during the day as a function of temperature and an increase in gain at the end of the day when temperature deceases again (compare with the temperature variation for the 2 d shown in Fig. 13). This will be further investigated after analyzing the temperature dependence for the whole data set.

Table 1Mean antenna gain from box scan data compared to the gain obtained from the operational monitoring. Antenna manufacturer gain is 45.4 (H) and 45.2 dB (V); see also Frech et al. (2013). The DRAO-data-based gain estimate is 45.4 dB.

Download Print Version | Download XLSX

In this section it is shown that the temperature sensitivity of S in the end can be attributed to the antenna assembly. The S variability as a function of temperature is initially evaluated based on over 2157 solar box scans acquired in early summer 2018. The temperature references for S, the radome temperature and the receiver temperature are taken. The radome temperature is the temperature in the volume surrounded by the radome. There is constant ventilation so that well-mixed temperature conditions can be expected. The RX temperature is measured in the receiver box close to the LNA which is mounted on a solid metal plate.

The scatter plots of S versus radome temperature and LNA temperature are shown in Fig. 13. A temperature span of 12 ^∘C is captured with this data set. It is apparent that the correlation of S and LNA temperature is better than the correlation with the radome temperature especially for radome temperatures between 22 and 24 ^∘C. The bin-wise median of S(T) (bin width is 1^∘) together with the first and third quartiles are shown in Fig. 13 with black lines. This implies that the origin of the S temperature dependence is maybe located in the receiver box, where the analog components for H and V obviously have a differential temperature dependence, though the correlation does not show cause and effect. This is examined more closely later. In Fig. 13b we fit a second-order polynomial Sfit(T)=a⋅T2+b⋅T+c to the −RX temperature data, with a=0.00208±0.00002 (dB∕T²), b=-0.132±0.0152 (dB∕T) and c=1.86±0.27 (dB). The fit to the curve resulting from the bin-averaged data is quite good.

Figure 13S scatter plot as a function of radome temperature (a) and LNA temperature (b). LNA temperature is a good proxy for the temperature variations within the receiver box. Also shown is the bin-wise-average (S(T) bin-wise avg; 1^∘ width and the first and third quartiles of all values within this bin) and a polynomial fit of second order (S_fit(T); see also text) of S versus LNA temperature (b). In total 2157 box scans are used for this analysis.

Download

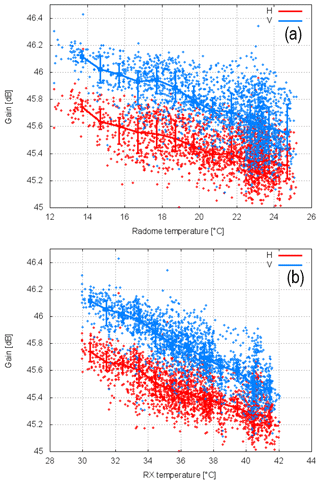
Figure 14Gain (H and V) scatter plot as a function of radome temperature (a) and LNA temperature (b). LNA temperature is a good proxy for the temperature variations within the receiver box. Also shown is the bin-wise-averaged gain (1^∘ width and the first and third quartiles of all gain values within this bin) as a function of temperature. In total 2157 box scans are used for this analysis.

Download

Similarly, Fig. 14 shows the dependence of solar-measured H and V gains on the receiver and radome temperatures. There is an obvious correlation of H and V gains with temperature. The best correlation appears if taking the receiver gain temperature as a reference (Fig. 14). If we use the bin-wise-averaged gain for H and V to compute the differential solar power S as g_H−g_V, we recover the curve shown in Fig. 13. The bin-wise-averaged gain and the resulting differential gain are shown in Fig. 15. We note the gains decrease by about 0.6 dB over a temperature difference of 10 ^∘C, and this indicates a temperature-dependent bias of the radar reflectivity factor Z. This temperature dependence is an additional contribution to the overall uncertainty in calibration, which is usually not considered in error assessments from manufacturers, where commonly engineering uncertainties in measuring the antenna gain and the transmit and receive losses among others are considered.

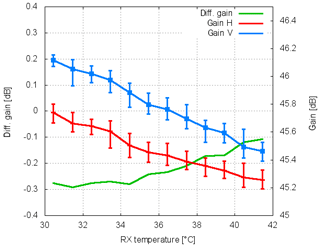
Figure 15Bin-wise-averaged gain (H and V; see Fig. 14) and computed as g_H−g_V versus receiver temperature.

Download

The differential power and gain temperature dependence appear to correlate best with the receiver temperature, and thus the LNAs and associated circuitry could be responsible for the seen temperature-dependent S observations. In order to evaluate the LNA gains, single-point calibration measurements are employed. With the one-point calibration, a test signal is injected into the receive path using a built-in test signal generator. Since the response of the digital receiver is linear, the receiver response for a test signal above noise level and below the receiver saturation can be used to determine dBZ0 and dBm0. In order to investigate the temperature sensitivity, the one-point calibration was carried out every 5 min as part of the operational 5 min scan cycle. A test signal can be coupled to the H and V receive paths at the antenna cross-guide coupler or at the LNA inside the receiver box. A 3 dB power splitter provides nearly equal power levels for both channels. A small power difference is inconsequential in evaluating the temperature sensitivity of the differential gain of the LNAs. The H and V signal path from the antenna cross-guide coupler includes a waveguide filter, a circulator and a TR limiter, which are all located behind the antenna outside the antenna-mounted receiver box and also manifest some dependence on temperature (see Fig. 2 in Frech et al., 2017). The temperature stability of the built-in TSG was characterized in a climate chamber where a very small temperature-dependent power output of the TSG of ±0.05 dB within a temperature range between 0 and 30 ^∘C was found. For proper calibration results, TSG losses between the coupler and the reference plane 1 (see Fig. 1) have been quantified using a network analyzer. The TSG losses between the antenna coupler and the reference plane 1 are measured as 77.96 dB (H) and 77.89 dB (V). If the TSG signal is injected at the LNA, the losses have been determined as 26.16 dB for both channels. Any observed temperature sensitivity based on the one-point calibration is therefore attributed to the respective receive paths. A data set with 2562 calibration results, where the TSG signal was coupled to the antenna cross-guide coupler, was acquired between 14 March 2019 and 23 March 2019. A second data set with 2810 calibration results, where the TSG signal was coupled to the LNAs, was acquired between 23 March and 5 April 2019.

The temperature-dependent measured power samples are shown in Fig. 16. The temperature dependence of both data sets is identical if either the receiver temperature or the radome temperature is used. Similar to the retrieved gain based on solar power, we find a 0.6 dB decrease over a comparable temperature range. The lower measured power levels in Fig. 16b are due to additional losses in the receive path. A remarkable feature of both independent data sets is the nonlinearity of the horizontal power sample at around 7 ^∘C (radome temperature) or 19 ^∘C (receiver temperature) with a ≈0.1 dB dip. This is not found for the power sample in V. The reason for this is still unknown. The corresponding differential power H–V is shown in Fig. 17. The nonlinearity in H leads to a −0.1 dB bias in differential power just for this specific part of the temperature range. For the rest of the temperature range only a very small temperature dependence in differential power up to 0.02 dB over a range of ≈16 ^∘C is found. The differential power based on power samples where the TSG is coupled to the antenna cross-guide coupler is on the order of 0.2 dB. This significantly larger difference compared to the other data set can be attributed not only to different insertion losses of the TR limiters and the circulators but also to the uncertainty in the measured TSG losses. But more importantly, there is again a very small temperature dependence of differential power.

Figure 16Bin-wise-averaged H and V power measurements based on the one-point calibration. Either the radome (T_rad) or the receiver box temperature (T_rx) is used as a reference. Shown is the median and the first and third quartiles of 1^∘ wide temperature bins. The results with the TSG coupled to the LNA (a) and to the antenna cross-guide coupler (b) are shown.

Download

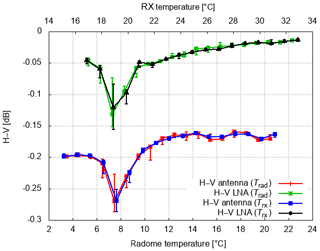
Figure 17Bin-wise-averaged differential power H–V based on the power samples from the one-point calibration. Either the radome (T_rad) or the receiver box temperature (T_rx) is used as a reference. Shown is the median and the first and third quartiles of 1^∘ wide temperature bins.

Download

This result is now compared to the S temperature dependence shown in Fig. 13. Even though the temperature ranges do not match precisely, some conclusions can be drawn. Based on solar box scan data a 0.2 dB increase in S over a temperature range of 12 ^∘C is found. The correlation with temperature is best if the receiver temperature is used to determine the mean temperature dependence of S. In contrast, the temperature sensitivity of differential power based on the one-point calibration indicates a substantially smaller temperature dependence with an increase of 0.02 dB or less over a comparable temperature range (excluding the anomalous behavior at 7 or 19 ^∘C). This behavior is essentially identical if either the radome temperature or the receiver temperature is used as a reference for the one-point-calibration results. Therefore, the temperature sensitivity observed in S can be attributed to the antenna assembly. There is one caveat however. There is a chance that the temperature dependence may be different in the two compared temperature ranges. This will be investigated in future measurements. This result is in agreement with the results in Hubbert (2017), who arrived at a similar conclusion. It is argued that the thermal expansion of the antenna assembly (including the struts) is responsible for a large part of the temperature sensitivity of differential power. This is still a matter of investigation, but initial simulations of antenna radiation patterns appear to support this conclusion. Details on the DWD antenna can be found in Frech et al. (2013).

Time series of the Z_DR calibration since the beginning of 2013 are available. From the beginning Z_DR was monitored using the birdbath scans and the solar interferences. How the Z_DR values agree for specific days has been shown in the previous section. The success of the automated Z_DR calibration procedure is discussed for all 17 radar sites up to September 2018 (Fig. 18). The methodology to determine Z_DR is described in Sect. 2. In Fig. 18 each data point represents a diurnal-averaged Z_DR value, which can only be determined if there are at least six birdbath scans available with precipitation. In total 16 646 diurnal-averaged Z_DR values (which corresponds to 45 years of data) are shown in Fig. 18. The corresponding total radar operation time amounts to 31 928 d (about 87 years). There is on average sufficient precipitation present on 52 % of the days. So for a given day, there is precipitation over the site for at least six birdbath scans. The median Z_DR is 0.0 dB, and the mean absolute deviation MAD is 0.029 dB (Wilks, 2011; Frech et al., 2017). This indicates that the method to update the Z_DR offset once a day appears sufficiently robust to provide a well-calibrated Z_DR. Further optimization is needed to eliminate the apparent outliers which may be caused by e.g., unwanted clutter contributions. Note that the diurnal variability in Z_DR due to, e.g., temperature, as discussed in this paper, is not captured by this automated procedure to determine the Z_DR offset. From one scan to another there might be deviations larger than 0.1 dB due to, e.g., temperature effects. The existence of larger Z_DR deviations is also indicated by the data in Fig. 18. In addition, the median Z_DR in nonoverlapping 5 d intervals is computed using the calibrated Z_DR data from the whole network. There appears to be no annual variation in Z_DR bias (Fig. 18), which is an indication that the method can be applied as a season-independent method without restricting the Z_DR offset analysis to liquid precipitation only.

Figure 18Radar-network-wide-calibrated Z_DR using birdbath data. Also shown is the median Z_DR over a 5 d interval from January 2013 until September 2018. There is no systematic annual variation in Z_DR, which implies that it is not necessary to distinguish the precipitation phase when calculating the Z_DR from birdbath data.

Download

Note that the radar systems have similar performance with respect to Z_DR bias (Fig. 19). There is only one system (radar Neuhaus, ID 9) which sticks out. But even this system is within the target corridor of ±0.1 dB.

Figure 19Z_DR bias of the 17 DWD radar sites, January 2013–August 2018. Shown is the median Z_DR and the first and third quartiles.

Download

The automated adjustment is well suited to correct for system drifts on a timescale longer than a day. This is shown in Fig. 20 for the Eisberg system. This is a case of an unusual TR limiter degradation of the Eisberg radar. TR limiters are usually located in front of the LNAs in order to protect the receiver from the transmit pulse (see Fig. 1). The transmit pulse causes the TR limiter to act as an open circuit for a short period of time called the recovery time. Typically an aging TR limiter shows an increase in its recovery time which causes an undesired attenuation of the received signal at ranges close to the radar but then extends in range. Here, the overall duration of attenuation of the TR limiter increased with time, which is illustrated by the fact that the S has a similar drift to the Z_DR determined from the birdbath scan. Over a time period of 9 months there is an increase in Z_DR bias of 3 dB. Since the increase in attenuation happens to be on a timescale longer than 1 d, the operational Z_DR offset adjustment is able to keep Z_DR within 0.2 dB (Z_DR-calibrated 90^∘, Fig. 20). After the replacement of the faulty TR limiter, the system operated reliably for about 3 months before the other limiter started to degrade in a similar way. Within about 9 months the Z_DR bias increased to −4 dB. The Z_DR bias is constant after the replacement of the TR limiter in October 2016. On the scale shown here, the Z_DR bias, as estimated from the birdbath measurement, is well approximated by S. Since S is only a function of the receiver electronics and the antenna and the birdbath measurements are a function of the receiver electronics, the antenna gains squared and the transmit power (Eqs. 6 and 17, respectively) it can be concluded that the transmit power ratio at the reference plane is relatively constant. Diurnal variations in S due to temperature are not seen in this daily average data.

Similar cases have been observed at other radar sites. The reason for this type of TR limiter failure is not known yet. This example nicely illustrates the benefit of using and combining different data sources to monitor and calibrate Z_DR.

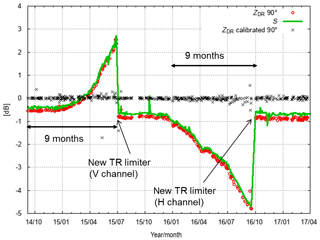
Figure 20TR limiter failure at the Eisberg radar. This illustrates the benefits of using solar monitoring and birdbath scans to assess biases in the receive path. Here, the combination of solar monitoring (solar power S) and birdbath analysis isolates the receive path as the source of the Z_DR problem. In addition we show the resulting calibrated Z_DR, where the calibration offset is determined from birdbath data automatically.

Download

Considering the classic aging of a TR limiter, a prototype of an automated analysis of the shape, and in particular the slope of the Z_DR profile has been implemented. Results are currently being analyzed. This approach will provide the height interval over which the mean Z_DR should be computed from the birdbath profile. Another promising approach to monitoring the classic aging of a TR limiter is the monitoring of clutter power in close vicinity of the radar (Mathijssen et al., 2018).

Full diurnal cycles of solar box scans have been acquired from four operational identical radar systems: Flechtdorf, FLD (27 June 2018); Neuheilenbach, NHB (24 July 2018); Boostedt, BOO (27 June 2018); and Hanover, HNR (24 July 2018). The solar box scans were evaluated similarly to the Hohenpeißenberg (MHP) box scans. The resulting diurnal series of gain estimates for H and V as a function of the solar azimuth position are shown in Fig. 21. In addition, the MHP gain estimates from 3 June 2018 are shown for comparison. Aside from the Flechtdort g_h, all gain estimates for both polarizations are within a 1 dB range and close to the nominal antenna gain of 45.4 dB. The diurnal variation in BOO shows a decrease in gain of up to 0.2 dB around a solar azimuth of 90 and 270^∘. The decrease is more pronounced for the vertical than the horizontal polarization. This feature can be related to the presence of lightning poles outside the radome. This decrease is not visible for the other operational systems because the azimuthal position of the lightning poles is such that the elevation of the sun during the measurements is too high so that the poles are not in the field of view of the antenna. Similar to MHP, a slight decrease in gain in the course of a day by 0.2 dB is also found for systems HNR, FLD and BOO. Diurnal temperature variations are the likely cause for this. The nominal antenna gain based on antenna pattern measurements is about 45.4 dB. A retrieved gain value, g_H, of close to 47 dB from FLD indicates a calibration error of 1.6 dB which can presumably be attributed to an erroneous characterization of the horizontal receive path.

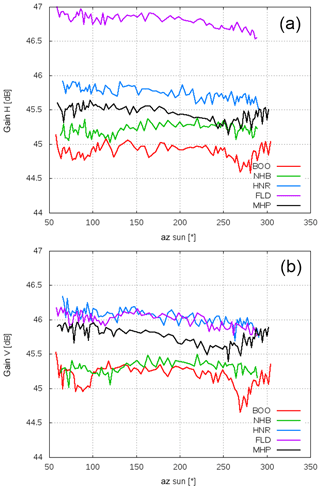
Figure 21Diurnal gain estimates as a function of solar azimuth from five radar systems, Boostedt (BOO), Flechtdorf (FLD), Hanover (HNR), Neuheilenbach (NHB) and Hohenpeißenberg (MHP). Shown is the gain of the horizontal receive channel (a) and vertical receive channel (b). The time series shown here are based on about 90 box scans that are available for each station.

Download

Data are available from the authors upon request.

MF carried out the data acquisition and performed the data analysis. JH wrote Sect. 2, provided the theoretical background in Sect. 2, and contributed to the analysis and interpretation of the results. MF prepared the manuscript.

The authors declare that they have no conflict of interest.

This paper was edited by Pavlos Kollias and reviewed by Martin Hagen and Richard Ice.