Mass Production of 2021 KMTNet Microlensing Planets II

Our approach to analyzing events is identical to that described in Section 3.1 of Paper I. Here, we present only the definitions of the parameter symbols, in conformity with standard practice. For more details, we refer the reader to Paper I.

All of the events in this paper can be analyzed to a first approximation as 1L1S events, which are characterized by three Paczyński (1986) parameters (t₀, u₀, t_E), i.e., the time of lens−source closest approach, the impact parameter (normalized to the Einstein radius, θ_E), and the Einstein radius crossing time

$$\begin{eqnarray}&&{t}_{{\rm{E}}}=\displaystyle \frac{{\theta }_{{\rm{E}}}}{{\mu }_{\mathrm{rel}}};\,\,\,\,{\theta }_{{\rm{E}}}=\sqrt{\kappa M{\pi }_{\mathrm{rel}}},\,\,\,\,\kappa \equiv \displaystyle \frac{4G}{{c}^{2}\,\mathrm{au}}\simeq 8.14\displaystyle \frac{\mathrm{mas}}{{M}_{\odot }}.\end{eqnarray} \tag{ 1 }$$

Here M is the mass of the lens, (π_rel, μ _rel) are the lens−source relative parallax and proper motion, μ_rel ≡ ∣ μ _rel∣, and nLmS means "n lenses and m sources."

A 2L1S model always requires at least three additional parameters (s, q, α), i.e., the separation (normalized to θ_E) and mass ratio of the two lens components, as well as the angle between the line connecting these and the direction of μ _rel. If there are finite-source effects due to the source approaching or crossing caustic structures that are generated by the lens, then one must also specify ρ ≡ θ_ast/θ_E, where θ_ast is the angular radius of the source.

For 1L2S models, which can generate featureless bumps that can be mistaken for 2L1S "planets" (Gaudi 1998), the minimal number of parameters is 6, including (t_0,1, t_0,2) and (u_0,1, u_0,2) for the two times of closest approach and impact parameters, respectively, t_E for the Einstein timescale, and q_F , i.e., the flux ratio of the two sources in the I band. In many cases, one or both of the two normalized source radii must be specified, ρ₁ = θ_ast,1/θ_E and ρ₂ = θ_ast,2/θ_E. More complex models involving orbital motion of the binary–source system may also be needed.

If the microlens parallax effect can be detected (or constrained), then one should include the microlens parallax vector (Gould 1992, 2000, 2004),

$$\begin{eqnarray}&&{{\boldsymbol{\pi }}}_{{\rm{E}}}=\displaystyle \frac{{\pi }_{\mathrm{rel}}}{{\theta }_{{\rm{E}}}}\,\displaystyle \frac{{{\boldsymbol{\mu }}}_{\mathrm{rel}}}{{\mu }_{\mathrm{rel}}},\end{eqnarray} \tag{ 2 }$$

which is normally expressed in equatorial coordinates π _E = (π_E,N , π_E,E ). In these cases, one usually must also fit, at least initially, for the first derivatives in time of the lens angular position, γ = [(ds/dt)/s, d α/dt], because π _E and γ can be correlated or even degenerate. In these cases, we restrict such fits to β < 0.8, where (An et al. 2002; Dong et al. 2009)

$$\begin{eqnarray}&&\beta \equiv \displaystyle \frac{\kappa {M}_{\odot }{\mathrm{yr}}^{2}}{8{\pi }^{2}}\displaystyle \frac{{\pi }_{{\rm{E}}}}{{\theta }_{{\rm{E}}}}{\gamma }^{2}{\left(\displaystyle \frac{s}{{\pi }_{{\rm{E}}}+{\pi }_{s}/{\theta }_{{\rm{E}}}}\right)}^{3},\end{eqnarray} \tag{ 3 }$$

and where π_S is the source parallax.

In our initial heuristic analyses, we often predict ${s}_{\pm }^{\dagger }$ and α from the morphology of the light curve (Hwang et al. 2022; Ryu et al. 2022),

$$\begin{eqnarray}&&{s}_{\pm }^{\dagger }=\displaystyle \frac{\sqrt{4+{u}_{\mathrm{anom}}}\pm {u}_{\mathrm{anom}}}{2};\,\,\,\tan \alpha =\displaystyle \frac{{u}_{0}}{{\tau }_{\mathrm{anom}}},\end{eqnarray} \tag{ 4 }$$

under the assumption that the anomaly occurs when the source crosses the binary axis. Here ${u}_{\mathrm{anom}}=\sqrt{{\tau }_{\mathrm{anom}}^{2}+{u}_{0}^{2}}$, τ_anom =(t_anom − t₀)/t_E, t_anom is the midpoint of the anomaly, and the "±" refers to major/minor-image perturbations. If there are two solutions, with normalized separation values s_±, as often occurs (see Zhang & Gaudi 2022 for a theoretical discussion of such degeneracies), we expect that the empirical quantity ${s}^{\dagger }=\sqrt{{s}_{+}{s}_{-}}$ (without subscript) will be approximately equal to the subscripted quantity from Equation (4).

Finally, we often report the "source self-crossing time," t_ast ≡ ρ t_E. We note that this is a derived quantity and is not fit independently.

Figure 1 shows an otherwise standard 1L1S light curve with Paczyński (1986) parameters (t₀, u₀, t_E) = (9349.32, 0.145, 91 days), punctuated by a 4.3-day double-horned profile, centered at t_anom = 9377.35. The double-horned profile is unusual in that it has a smooth bump in the middle, which is almost certainly generated by the source approaching an interior wall of the caustic.

Zoom In Zoom Out Reset image size

3.2.1. Heuristic Analysis

These parameters imply τ_anom = 0.308, u_anom = 0.340, and thus

$$\begin{eqnarray}&&\alpha =25^\circ ;\,\,\,\,{s}_{+}^{\dagger }=1.18.\end{eqnarray} \tag{ 5 }$$

Because the anomaly is clearly due to the source entering and leaving the caustic, we do not expect a degeneracy in s. Rather, we expect $s\simeq {s}_{+}^{\dagger }$.

3.2.2. Static Analysis

The grid search on the (s, q) plane returns only one solution, whose refinement with all parameters set free is shown in Table 2. We find that α and s are as expected, while $\mathrm{log}q=-3.3$ indicates a Saturn mass ratio planet. The caustic entrance and exit are both well covered, yielding a ∼8% measurement of a relatively low value of ρ = 3.9 × 10⁻⁴. We will see in Section 4.1 that this implies a large value of θ_E ∼ 0.64 mas, and so a relatively nearby lens π_rel ∼0.05 mas/(M/M_⊙) and thus a relatively large microlens parallax π_E ∼ 0.08(π_rel/0.05 mas). Together with the relatively long timescale and the fact that the anomaly has three peaks (An & Gould 2001), this encourages us to search for microlens parallax solutions, in spite of the relatively faint source, I_S,KMTC01 ∼ 21.6.

Table 2. Microlens Parameters for KMT-2021-BLG-0712

		Parallax Models
Parameters	Standard	u0 > 0	u0 < 0
χ2/dof	4263.666/4264	4224.244/4260	4222.276/4260
t0 − 2,459,340	9.317 ± 0.115	9.954 ± 0.144	10.068 ± 0.129
u0	0.147 ± 0.002	0.145 ± 0.002	−0.144 ± 0.002
tE (days)	90.865 ± 0.951	88.503 ± 1.442	100.583 ± 2.039
s	1.196 ± 0.002	${1.196}_{-0.012}^{+0.016}$	1.206 ± 0.020
q (10−4)	4.751 ± 0.141	${5.121}_{-0.654}^{+0.998}$	5.653 ± 1.157
log q (mean)	−3.323 ± 0.014	−3.285 ± 0.062	−3.251 ± 0.079
α (rad)	0.467 ± 0.004	${0.452}_{-0.034}^{+0.027}$	5.788 ± 0.039
ρ (10−4)	3.903 ± 0.330	4.010 ± 0.449	4.221 ± 0.551
πE,N	⋯	0.425 ± 0.104	0.701 ± 0.111
πE,E	⋯	−0.047 ± 0.034	−0.204 ± 0.042
ds/dt (yr−1)	⋯	${0.008}_{-0.199}^{+0.152}$	−0.149 ± 0.262
d α/dt (yr−1)	⋯	$-{0.688}_{-0.321}^{+0.415}$	−0.902 ± 0.602
IS [KMTC01,pySIS]	21.618 ± 0.013	21.644 ± 0.017	21.653 ± 0.018
IB [KMTC01,pySIS]	18.676 ± 0.001	18.672 ± 0.002	18.673 ± 0.002
tast (hr)	0.851 ± 0.069	0.852 ± 0.092	1.019 ± 0.138

Download table as:  ASCIITypeset image

3.2.3. Parallax Analysis

As is almost always the case (except for some extremely long events), there are two parallax solutions, which are summarized in Table 2 and illustrated in Figure 2. As described in Section 3.1, we simultaneously fit for the first derivatives of the planet position due to its orbital motion γ = [(ds/dt)/s, d α/dt]. While Table 2 gives π _E in standard equatorial coordinates, it is also useful to present these solutions in terms of the principal axes of the error ellipses,

$$\begin{eqnarray}\begin{array}{rcl}({\pi }_{{\rm{E}},\parallel },{\pi }_{{\rm{E}},\perp },\psi ) & = & (+0.123\pm 0.028,0.40\pm 0.11,280.4^\circ )\\ & & \times \,[{u}_{0}\,\gt \,0]\end{array}\end{eqnarray} \tag{ 6 }$$

and

$$\begin{eqnarray}\begin{array}{rcl}({\pi }_{{\rm{E}},\parallel },{\pi }_{{\rm{E}},\perp },\psi ) & = & (+0.033\pm 0.028,0.72\pm 0.14,256.4^\circ )\\ & & \times \,[{u}_{0}\lt 0].\end{array}\end{eqnarray} \tag{ 7 }$$

Here π_E,∥ (so called because, for short events, it is approximately parallel to the projected position of the Sun) is the minor axis of the error ellipse, π_E,⊥ is the major axis, and ψ is the angle of the minor axis, measured north through east. In line with the sign conventions of Figure 3 of Park et al. (2004; keeping in mind that MOA-2003-BLG-037 peaked after opposition while KMT-2021-BLG-0712 peaked before opposition), π_E,∥ is approximately west and π_E,⊥ is approximately north. Note that the actual projected orientation of Earth relative to the Sun at the peak is ψ_⊙ = 2810. Because the full width at half maximum of magnification versus time, ${t}_{\mathrm{FWHM}}\simeq \sqrt{12}{u}_{0}{t}_{{\rm{E}}}\sim 45\,$ days, covers almost a radian of Earth's orbit, the "short event" approximation (Smith et al. 2003; Gould 2004; Park et al. 2004) is not expected to yield a precise characterization.

Zoom In Zoom Out Reset image size

We find, from fitting the event (with the anomaly removed) to a point-lens model with parallax, that the presence of the anomaly reduces the axes of the error ellipses for the two solutions from (0.030:0.45) to (0.028:0.11) and from (0.032:0.39) to (0.028:0.14), in particular, reducing the aspect ratios by factors of 3.8 and 2.4 for the two cases. This confirms the important role of the relatively complex caustic features in improving the parallax measurement.

Figure 3 shows an otherwise approximately standard 1L1S light curve with parameters (t₀, u₀, t_E) = (9354.1, 0.060, 16 days), punctuated by a sharp bump, which erupts suddenly at 9360.65 and then peaks Δt_rise = 4 hr later at t_anom =9360.82. This is almost certainly a caustic entrance, although there is no obvious caustic exit.

Zoom In Zoom Out Reset image size

3.3.1. Heuristic Analysis

These parameters imply τ_anom = 0.420, u_anom = 0.424, and thus

$$\begin{eqnarray}&&\alpha =188\buildrel{\circ}\over{.} 1;\,\,\,\,{s}_{+}^{\dagger }=1.23.\,\,\,\,{s}_{-}^{\dagger }=0.81.\end{eqnarray} \tag{ 8 }$$

Note that because the nature of the caustic entrance is unclear, we report both ${s}_{+}^{\dagger }$ and ${s}_{-}^{\dagger }$. Moreover, because u_anom ∼ 0.4 is large, this is a planetary caustic crossing, so we do not expect a degeneracy in s. Rather, for a major-image caustic, we expect $s\simeq {s}_{+}^{\dagger }$, while for a minor-image caustic, we expect a less precise $s\sim {s}_{-}^{\dagger }$ because the caustic would not lie on the binary axis.

3.3.2. Static Analysis

3.3.3. Parallax Analysis

3.3.4. Xallarap Analysis

As a matter of due diligence, we also check whether the light-curve features that were judged to be due to "systematics" in the previous section are, in fact, due to another real physical phenomenon: xallarap. The term "xallarap" refers to light-curve distortions induced by orbital motion of the source around an unseen companion (Griest & Hu 1992; Han & Gould 1997). Xallarap can be an important test of the reality of a parallax signal because any light curve that can be fit by parallax can be fit equally well by xallarap, with source motion parameters that mimic those of Earth. Then, if these xallarap fit parameters do closely mimic Earth's, it would be extraordinarily improbable that these were due to source motion rather than Earth motion, while if they deviated strongly from Earth's parameters, it would be a sign that the light-curve distortion was mainly due to xallarap or, perhaps, some systematics (Poindexter et al. 2005).

Compared to a standard seven-parameter 2L1S fit, xallarap has five additional parameters (for the simplest xallarap model, i.e., a circular orbit): (P, α_s , δ_s , ξ ), where ξ = (ξ_N , ξ_E ). Here P is the orbital period of the source, ξ is the amplitude and orientation (at t₀) of the source orbit scaled to θ_E, and (α_s , δ_s ) give the orientation of the orbital plane, with 90° – α being the orbital inclination. This parameterization is chosen so that if the xallarap signal is actually due to parallax, then one will find (within errors) that P = 1 yr, ξ = π _E, and (α_s , δ_s ) will be the ecliptic coordinates of the event.

We find a best xallarap fit with P = 0.4 yr, ξ = (−1.32, + 2.20), (α, δ) = (190°, 6°), and with the other seven parameters being similar to those of the 2L1S standard solution.

From Kepler's third law (and Newton's third law), one finds

$$\begin{eqnarray}&&\displaystyle \frac{{Q}^{3}}{{\left(1+Q\right)}^{2}}=\displaystyle \frac{{\left({a}_{S}/\mathrm{au}\right)}^{3}}{({M}_{S}/{M}_{\odot }){\left(P/\mathrm{yr}\right)}^{2}}\to 5975,\end{eqnarray} \tag{ 9 }$$

where Q = M_C /M_S is the ratio of the companion mass to that of the source, a_S = ξ D_S θ_E = ξ D_S θ_ast/ρ → 9.85 au is the orbital radius of the source, and we have adopted M_S = 1 M_⊙, D_S = 8 kpc, θ_ast = 1.2 μas from Section 4 and ρ = 2.5 × 10⁻³ from the xallarap fit. Hence, in this solution, the companion mass would be M_C = 6000 M_⊙. Hence, we reject this solution as spurious, i.e., due to the same systematics that generated the improbable parallax solution.

Figure 4 shows an approximately standard 1L1S light curve with parameters (t₀, u₀, t_E) = (9482.2, 0.08, 43 days), but with two major features superposed: a poorly sampled caustic feature, centered at ∼9486, lasting 1.5–2 days, and a roughly 2-day, roughly symmetric spike, peaking at 9493.9. The sparse coverage is primarily due to the fact that these anomalies occurred near the end of the microlensing season, when the field was visible only about 3 hr per night from each site, and partly due to episodes of adverse weather.

Zoom In Zoom Out Reset image size

The first (i.e., caustic) structure implies that there must be a second lens. If the system is not more complicated than this, i.e., it is 2L1S, then the presence of two anomalies at τ₁ ∼ +0.09 and τ₂ ∼ +0.29 after peak almost certainly implies a very large resonant caustic. In principle, however, such multiple anomalies might require more complex systems, such as 3L1S.

3.4.1. Heuristic Analysis

Within the 2L1S framework, t_anom = 9493.9, i.e., τ_anom = τ₂ = 0.27, and u_anom = 0.29, so

$$\begin{eqnarray}&&\alpha =16\buildrel{\circ}\over{.} 5;\quad {s}_{+}^{\dagger }=1.16.\end{eqnarray} \tag{ 10 }$$

3.4.2. Static Analysis

The grid search yields only one solution, whose refined parameters are given in Table 4. Note that while the heuristic α prediction was approximately correct, the heuristic ${s}_{+}^{\dagger }$ (combined with s = 1.055 from Table 4), predicts a second solution at ${s}_{\mathrm{inner}}={({s}_{+}^{\dagger })}^{2}/{s}_{\mathrm{outer}}=1.28$. Such solutions can generate a cusp-approach spike as the source passes over the ridge between the central and planetary caustics, but the central caustic is not large enough to induce the first caustic anomaly that is seen in the light curve. Hence, there is no degeneracy.

Table 4. Microlens Parameters for KMT-2021-BLG-2478

		Parallax + Orbital Motion Models
Parameters	Standard	u0 > 0	u0 < 0
χ2/dof	11327.812/11268	11263.895/11265	11263.879/11265
t0 − 2,459,480	2.104 ± 0.017	2.162 ± 0.026	2.156 ± 0.026
u0	0.078 ± 0.001	0.089 ± 0.002	−0.088 ± 0.002
tE (days)	43.111 ± 0.433	37.907 ± 0.660	38.049 ± 0.702
s	1.055 ± 0.001	1.057 ± 0.001	1.056 ± 0.001
q (10−3)	3.109 ± 0.101	4.327 ± 0.304	4.206 ± 0.305
log q (mean)	−2.507 ± 0.014	−2.365 ± 0.031	−2.376 ± 0.032
α (rad)	0.276 ± 0.001	0.261 ± 0.010	6.020 ± 0.010
ρ (10−4)	4.504 ± 2.262	${8.545}_{-3.938}^{+2.967}$	${9.271}_{-3.567}^{+2.753}$
πE,N	⋯	0.101 ± 0.272	0.078 ± 0.248
πE,E	⋯	0.355 ± 0.052	0.339 ± 0.053
ds/dt (yr−1)	⋯	0.553 ± 0.120	0.549 ± 0.116
d α/dt (yr−1)	⋯	0.589 ± 0.579	−0.113 ± 0.552
IS [KMTC(01),pySIS]	20.766 ± 0.014	20.607 ± 0.021	20.617 ± 0.022
IB [KMTC(01),pySIS]	18.063 ± 0.001	18.077 ± 0.002	18.077 ± 0.002
tast (hr)	0.466 ± 0.235	${0.777}_{-0.359}^{+0.273}$	${0.847}_{-0.327}^{+0.255}$
β (θast ≡ 0.5μas)	⋯	${0.226}_{-0.102}^{+0.203}$	${0.161}_{-0.069}^{+0.126}$

Download table as:  ASCIITypeset image

As with KMT-2021-BLG-0909, this planet has a super-Jovian mass ratio.

The Markov Chain Monte Carlo (MCMC) constraints on ρ are not adequately summarized by the median and 68-percentile format of Table 4. In Section 4.3, we will show that the best fit is ρ ∼ 1.1 × 10⁻³ and the 3σ upper limit is ρ < 1.6 × 10⁻³, while θ_ast ∼ 0.5 μas. Hence, these ρ values would imply θ_E = 0.45 mas and θ_E > 0.3 mas, respectively. On the other hand, even extremely low values, ρ ≪ 10⁻³, are consistent with the data at Δχ² < 5. Hence, we must at least consider the possibility of very large ${\theta }_{{\rm{E}}}=\sqrt{\kappa M{\pi }_{\mathrm{rel}}}$ and hence very nearby and/or very massive lenses. The former would imply a large and hence potentially measurable microlens parallax π_E. As mentioned in Section 3.2.2, the complex caustic structure, spanning a significant fraction of t_E, greatly enhances the prospects for making such a measurement.

3.4.3. Parallax Analysis

Including π _E and γ improves the fit by Δχ² = 64, with the u₀ > 0 and u₀ < 0 being almost perfectly degenerate. See Table 4 for the parameters of these fits and Figure 5 for the χ² distribution on the π _E plane. The most important aspect of these fits is that π_E ∼ 0.37 ± 0.06 is indeed large, implying M = θ_E/κ π_E ∼ 0.18 M_⊙ and D_L = 1/(π_E θ_E + π_S ) ∼ 3.4 kpc. However, because the errors, particularly in ρ (and hence θ_E), are large, proper estimates will require a Bayesian analysis. See Section 5.3.

Zoom In Zoom Out Reset image size

3.4.4. 3L1S and 2L2S Analyses

Figure 6 shows an otherwise standard 1L1S light curve with parameters (t₀, u₀, t_E) = (9375.8, 0.11, 35 days), punctuated by a sharp spike at t_anom = 9373.4, i.e., 2.3 days before peak.

Zoom In Zoom Out Reset image size

3.5.1. Heuristic Analysis

These parameters imply τ_anom = 0.07, u_anom = 0.13, and so

$$\begin{eqnarray}&&\alpha =122^\circ ;\quad {s}_{+}^{\dagger }=1.067.\end{eqnarray} \tag{ 11 }$$

3.5.2. Static Analysis

Somewhat surprisingly, the grid search returns six local minima. After refinement, we reject two of these because they have high Δχ² = 69 and 109 and, moreover, have poor fits by eye. However, we briefly note that both have relatively high mass ratios $\mathrm{log}q\sim -1.75$, and for both the spike arises from an off-axis cusp approach to a resonant caustic. While these models are certainly not correct, they emphasize the importance of making a systematic search of parameter space because the overall appearance of the models is not qualitatively different from the observed light curve.

The remaining four models are shown in Figure 6, with the corresponding geometries shown in Figure 7, while their refined parameters are given in Table 5. Locals 1 and 2 constitute an inner/outer degeneracy with s^† = 1.068, while Locals 3 and 4 constitute a second inner/outer degeneracy with s^† = 1.066, both in excellent agreement with Equation (11). This again emphasizes the importance of a systematic search. Because Locals 3 and 4 are each disfavored by Δχ² > 10, we consider that these solutions are excluded. Nevertheless, it is notable that these two pairs of solutions differ in q by more than a factor of 2.

Zoom In Zoom Out Reset image size

Table 5. Microlens Parameters for KMT-2021-BLG-1105

Parameters	Local 1	Local 2	Local 3	Local 4
χ2/dof	1784.854/1785	1788.955/1785	1795.837/1785	1806.507/1785
t0 − 2,459,370	5.826 ± 0.036	5.856 ± 0.036	5.711 ± 0.036	5.780 ± 0.035
u0	0.109 ± 0.007	0.107 ± 0.007	0.107 ± 0.007	0.101 ± 0.005
tE (days)	34.965 ± 1.919	${35.374}_{-1.739}^{+2.264}$	34.425 ± 1.985	${36.248}_{-1.488}^{+1.887}$
s	1.214 ± 0.008	0.939 ± 0.008	1.265 ± 0.013	0.899 ± 0.010
q (10−3)	1.984 ± 0.200	1.934 ± 0.191	4.939 ± 0.694	4.573 ± 0.614
log q (mean)	−2.703 ± 0.044	−2.714 ± 0.043	−2.308 ± 0.062	−2.341 ± 0.058
α (rad)	2.140 ± 0.009	2.148 ± 0.010	2.136 ± 0.010	2.152 ± 0.009
ρ (10−3)	<1.3	<1.3	<1.3	<1.3
IS [KMTC,pySIS]	21.233 ± 0.074	${21.258}_{-0.070}^{+0.085}$	21.207 ± 0.080	21.294 ± 0.062
IB [KMTC,pySIS]	18.614 ± 0.006	18.612 ± 0.006	18.616 ± 0.006	18.609 ± 0.004
tast (hr)	<1.1	<1.1	<1.1	<1.1

Download table as:  ASCIITypeset image

This is another super-Jovian mass ratio planet, $\mathrm{log}q=-2.7$.

We note that while ρ is not measured, the constraint, ρ < 0.0013, at 2.5σ, corresponding to t_ast < 1.1 hr, is strong enough to play a significant role. That is, in Section 4.4 we will show that θ_ast = 0.5 μas, implying μ_rel = θ_ast/t_ast > 4 mas yr⁻¹, which excludes a significant part of proper-motion parameter space. Hence, when we incorporate the ρ constraint to estimate the physical parameters of the system in Section 5.4, we apply the full χ²(ρ) envelope function, rather than a simple limit. For the moment, we simply note that even the 1σ "limit" corresponds to μ_rel > 6.5 mas yr⁻¹, and so it still leaves a substantial range of values that are well populated by Galactic models. This fact will become relevant in Section 3.5.3.

Due to the faintness of the source and the lack of complex anomaly structures, we do not attempt a parallax analysis.

3.5.3. Binary–Source Analysis

As with all bump-like anomalies that lack complex or caustic-crossing features, we must check whether the anomaly can be produced by a second source (1L2S) rather than a second lens (2L1S). The results are shown in Table 6.

Table 6. 1L2S for KMT-2021-BLG-1105

Parameters	1L2S	ρ2 = 0.001	ρ2 = 0.0
χ2/dof	1790.359/1785	1800.137/1786	1803.805/1786
t0,1 − 2459370	6.162 ± 0.041	6.151 ± 0.041	6.192 ± 0.043
u0,1	0.105 ± 0.010	${0.077}_{-0.005}^{+0.007}$	0.103 ± 0.010
tE (days)	37.787 ± 3.115	49.293 ± 3.400	38.450 ± 3.202
t0,2 − 2459370	3.389 ± 0.002	${3.388}_{-0.004}^{+0.003}$	3.381 ± 0.004
u0,2 (10−3)	0.000 ± 0.313	0.025 ± 0.322	0.654 ± 0.101
ρ2 (10−3)	1.416 ± 0.139	1	⋯
qF (10−2)	1.021 ± 0.055	1.065 ± 0.072	1.186 ± 0.088
IS [KMTC,pySIS]	21.355 ± 0.113	${21.703}_{-0.098}^{+0.080}$	21.381 ± 0.113
IB [KMTC,pySIS]	18.605 ± 0.007	${18.586}_{-0.003}^{+0.005}$	18.603 ± 0.007
IS,2 [KMTC,pySIS]	26.344 ± 0.103	${26.657}_{-0.106}^{+0.065}$	26.211 ± 0.111
tast,2 (hr)	1.284 ± 0.068	1.183 ± 0.082	⋯

Download table as:  ASCIITypeset image

There are two main features to note about this solution. First, while the χ² difference, Δχ² = χ²(1L2S) − χ²(2L1S) = 5.5, favors the 2L1S solution, it is not large enough, by itself, to definitively rule out the 1L2S solution.

Second, the value of the second-source self-crossing time, t_ast,2 = 1.28 hr, is well measured in this model (contrary to 2L1S), with just a 5% error. At first sight, this value appears to be very "typical" of historic measurements of t_ast for dwarf-star sources in microlensing events. However, in this instance, the source is about 100 times fainter than typical cases, I_S,2 = 26.3. We will show in Section 4.4 that this implies θ_ast,2 = 0.169 μas and thus μ_rel = θ_ast,2/t_ast,2 = 1.16 mas yr⁻¹. Only a fraction of $p\lt {({\mu }_{\mathrm{rel}}/{\sigma }_{\mu })}^{3}/6\sqrt{\pi }\to 0.006$ microlensing events will have such low proper motions (e.g., Gould et al. 2021, 2022a). Here, we have approximated the bulge proper-motion distribution as an isotropic Gaussian, with σ_μ = 2.9 mas yr⁻¹. For example, in a systematic study of 30 1L1S events with finite-source effects (thus permitting μ_rel measurements), which was sensitive to μ_rel ≥ 1.0 mas yr⁻¹, Gould et al. (2022b) found that the slowest (KMT-2019-BLG-0527) had μ_rel =1.45 mas yr⁻¹, i.e., σ_μ /2. See their Figure 5. Thus, the combination of the Δχ² preference discussed above with this kinematic argument overwhelmingly favors the 2L1S (i.e., planetary) interpretation. ¹¹

For completeness, we remark that because the second source would be very red, the 1L2S model predicts that the bump anomaly would be much less pronounced in the V band than the I band. See, e.g., Hwang et al. (2018) for a practical example. Unfortunately, however, there are no V-band data during the anomaly.

Because the interpretation of the event rests heavily on the kinematic argument, we must also consider the possibility that this argument can be evaded (at some cost in χ²) by solutions with much smaller ρ. We first check that the 1σ error bar on ρ₂ shown in Table 6 is actually representative of the χ² surface out to 3σ by fixing ρ₂ at various values. We find that it is. See Table 6 for an example. Next, we search for solutions that are away from this local minimum by enforcing ρ₂ = 0. We find that there is such a solution, but it is disfavored by Δχ² = 13.4. See Table 6. Thus, while this solution avoids the proper-motion constraint, it increases the total χ² difference to Δχ² =χ²(1L2S) − χ²(2L1S) = 19. This would be high enough to decisively reject 1L2S were we to adopt the low-ρ₂ solution.

Moreover, there is an additional statistical argument against the 1L2S solution. From the Local 1 panel of Figure 7, it is clear that there is a range of "x," i.e., u_x , of about 0.15 Einstein radii that would generate a qualitatively similar non-caustic-crossing bump. However, the 1L2S solution requires the source to cross the face of the second source, which has a probability of p = 2ρ₂ ≃ 0.003, i.e., about 50 times smaller. To fully evaluate this relative probability, we would have to consider the relative probabilities of the presence of a lens planetary companion, compared to a source M-dwarf companion, which we do not attempt here because it is unnecessary to make the basic argument. Nevertheless, it is clear that the 1L2S solution requires some fine-tuning.

While we cannot absolutely rule out the 1L2S solution, the formal probability that it is correct is about p ∼ 1/400. Hence, this planet should be accepted as genuine. We note that its reality can be definitively tested at first adaptive optics (AO) light on next-generation (30 m) telescopes, roughly in 2030, i.e., Δt = 9 yr after the event. If, as anticipated, the 2L1S model is correct, then the source and lens will be separated by Δθ = μ_relΔt ≳ 36 mas, so they will be easily resolved. On the other hand, if the 1L2S model were correct, then the separation would be Δθ ∼ 9 mas yr⁻¹, which would probably be too small to resolve, but even if resolved, it would provide a measurement that was consistent with 1L2S but not with 2L1S.

Before leaving the issue of 1L2S models, we note that, as a matter of "due diligence," we explored 1L2S models in which finite-source effects were permitted for both the primary and secondary sources, even though such effects are extremely unlikely for the primary, a priori, because t_eff ≡ u₀ t_E ∼ 4 days is extremely long relative to the typical self-crossing time of dwarf stars, t_ast ∼ 1 hr. Surprisingly, we did indeed find such solutions with Δχ² ∼ −6 relative to the solution reported in Table 6 (and hence comparable χ² to the 2L1S solution). However, these had (ρ₁, ρ₂) ≃ (0.2, 0.002), which would imply grossly inconsistent estimates for θ_E = θ_ast/ρ of 2.5 μas versus 85 μas. Hence, it is unphysical. The χ² improvement could be a purely statistical fluctuation (p = 0.05), or it could be due to low-level systematics in the photometry. In any case, we reject this solution.

Finally, we remark that this event was included in the present study only because our "mass production" project aims to document all 2021 events with viable planetary solutions, in the spirit pioneered by Gould et al. (2022a) and Jung et al. (2022) for 2018 events, irrespective of whether such planetary solutions are decisively preferred. Our initial assessment, based on detailed modeling of TLC reductions, was that its interpretation was ambiguous, and thus it would not enter planetary catalogs. It was only in the course of comprehensively evaluating all the evidence that we concluded that the planetary solution is decisively favored.

There are two issues related to the source that require some care for this event. First, the source color shown in Table 7, (V − I)_S,0 = 0.69 ± 0.06, is unusually blue given that the source lies ΔI = 5.9 mag below the clump. If the source were a typical bulge star of this brightness, we would expect (V − I)_S,0 ∼ 1.1, based on Hubble Space Telescope (HST) images of Baade's window taken by Holtzman et al. (1998). Logically, there are three possibilities: our color measurement is incorrect, the source lies well behind the bulge and thus is much more luminous (and so bluer) than a bulge star of similar brightness, or the source is atypical, e.g., has much lower metallicity than typical bulge stars. The first of these explanations is the only one of direct concern here: if the color and magnitude of the source are correctly measured, regardless of the exact cause of it being so blue, then the derived θ_ast will also be correct.

We therefore check the color determination as follows: The color and magnitude reported in Table 7 are based on the KMTC41 data set. We repeat the calculation using the KMTC01 data set, which is composed of a completely independent series of observations. While these observations are made with the same (KMTC) telescope, the observational times are different, and the positions on the focal plane are offset by ${8}^{{\prime} }$. However, the best-fit color is the same to within 0.01 mag. Neither of the other two explanations appears likely a priori. To be sufficiently more luminous to account for the color discrepancy, the source should be roughly a factor of 2 more distant than the bulge, which would place it almost 1 kpc below the Galactic plane. While there are certainly some stars at this height and this Galactocentric radius (i.e., similar to that of the Sun), they are relatively rare. Extremely metal-poor stars in the bulge are likewise rare.

Despite the low prior likelihood of either of these two options, they are not unphysical, and hence we adopt the measured color, and hence the value of θ_ast = 0.255 μas given in Table 7, and we thereby derive

$$\begin{eqnarray}\begin{array}{rcl}{\theta }_{{\rm{E}}} & = & 0.604\pm 0.095\,\mathrm{mas};\,\,{\mu }_{\mathrm{rel}}=2.19\pm 0.34\,\mathrm{mas}\,{\mathrm{yr}}^{-1},\\ & & \times \,({u}_{0}\lt 0)\end{array}\end{eqnarray} \tag{ 15 }$$

and

$$\begin{eqnarray}\begin{array}{rcl}{\theta }_{{\rm{E}}} & = & 0.636\pm 0.091\,\mathrm{mas};\,\,{\mu }_{\mathrm{rel}}=2.62\pm 0.37\,\mathrm{mas}\,{\mathrm{yr}}^{-1},\\ & & \times \,({u}_{0}\,\gt \,0).\end{array}\end{eqnarray} \tag{ 16 }$$

The second issue that requires some care is the location of the blend relative to the source. If these were closely aligned, it would argue for the blend being associated with the event, being either the lens itself or a companion to the lens or the source.

In the KMTC41 pyDIA analysis, the baseline object appears to lie Δ θ (N, E) = (170, 145) mas northeast of the source. The issue that requires care is that there is another, slightly brighter star that lies 1'' northwest of the baseline object, which could in principle corrupt the astrometry of the baseline object. (The position of the source is determined from difference images, for which no such issues arise.) We conduct two tests. First, we repeat the analysis using the KMTC01 observations and find almost exactly the same result. Second, we find, after transforming coordinates to the OGLE-III system, that the offset is qualitatively similar: Δ θ (N, E) = (80, 250) mas. Note that because the epoch of the OGLE-III data is 15 yr earlier, we expect offsets of order 50 mas in each direction, in addition to normal measurement errors. The blend is 0.12 mag bluer and 3.38 mag fainter than the clump (see Figure 8), and it is therefore likely to be a bulge subgiant. We conclude that it is most likely not related to the event. The lens must be fainter than the blend, but because the two are separated by just 220 mas, we cannot place more stringent constraints on the lens light than this.

Due to high extinction, A_I ∼ 4, the source is too faint in the V band to measure the source color from the light curve. In such cases, one generally estimates the source color based on its offset in the I band from the centroid of the red clump. As often happens for such heavily reddened fields, it is difficult to precisely locate the red clump on the pyDIA (or, when available, OGLE-III) CMD because even red clump stars are near or below the measurement threshold in the V band. In the present case, we find that the red clump is detectable on the pyDIA CMD, but its centroid cannot be reliably determined because the lower part of the clump merges into the background noise of the diagram.

Therefore, we measure the clump centroid on an [(I − K), I] CMD, which we construct by matching pyDIA I-band photometry with K-band photometry from the VVV catalog (Minniti et al. 2017). See Figure 8. To estimate the color, we first find the offset from the clump ΔI = I_S − I_cl = 2.85 ± 0.08. See Table 7. If the source were exactly at the mean distance of the clump, it would therefore have an absolute magnitude, M_I = 2.73. In fact, it is more likely to be toward the back of the bulge (because it must be behind the lens), so a plausible range of possibilities is 2.2 ≲ M_I ≲ 2.9. In this range, the source could be almost anywhere along the turnoff/subgiant branch. To account for this, we adopt a uniform distribution, 0.60 <(V − I)_S,0 < 1.00, which we summarize as a 1σ range of (V − I)_S,0 = 0.80 ± 0.12. This source position is illustrated in Figure 8 by transforming from (V − I) to (I − K) using the relations of Bessell & Brett (1988). These values lead to estimates of

$$\begin{eqnarray}&&{\theta }_{{\rm{E}}}=0.362\pm 0.049\,\mathrm{mas};\,\,\,\,{\mu }_{\mathrm{rel}}=8.24\pm 1.11\,\mathrm{mas}\,{\mathrm{yr}}^{-1}.\end{eqnarray} \tag{ 17 }$$

Also shown in the CMD is the position of the blended light, which is a bright giant that is more than 1 mag above the clump. We find that this star is displaced by 0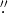73 from the source toward the southwest. This bright star is almost certainly not associated with the event, but it prevents us from placing any useful limits on the lens light.

For completeness, we note that the coordinates shown for this event in Table 1 are, as usual, those of the nearest catalog star, namely the bright giant just discussed. However, these differ from the coordinates shown on the KMT web page, which are about 1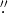5 yet farther south. When the event was originally triggered by AlertFinder (Kim et al. 2018b), it was identified with this more southerly catalog star. One day later, it was again triggered, this time by the closer (bright) catalog star, but our standard procedures enforce maintaining the coordinates of the original announcement on the web page to avoid confusion.

The source star, whose parameters are given in Table 7 and whose CMD position is shown in Figure 8, lies 4.5 mag below the clump and is about 0.07 ± 0.06 mag redder than the Sun. That is, it is a bulge mid-G dwarf. Unfortunately, as discussed in Section 3.4, we have only a χ²-envelope constraint on ρ, rather than a precise measurement. See Figure 9. For the present, we therefore give the estimates of θ_E and μ_rel scaled to that section's best estimate,

$$\begin{eqnarray}\begin{array}{rcl}{\theta }_{{\rm{E}}} & = & \displaystyle \frac{1.1\,\times \,{10}^{-3}}{\rho }(0.45\pm 0.04)\,\mathrm{mas}\\ {\mu }_{\mathrm{rel}} & = & \displaystyle \frac{1.1\,\times \,{10}^{-3}}{\rho }(4.3\pm 0.4)\,\mathrm{mas}\,{\mathrm{yr}}^{-1}.\end{array}\end{eqnarray} \tag{ 18 }$$

Zoom In Zoom Out Reset image size

Figure 8 shows the location of the blended light, which is 2.35 mag brighter than the source and of similar color. We find that the source is displaced from the baseline object by Δθ = 220 mas to the southeast. It is consistent with being a bulge turnoff/subgiant star and thus could in principle be a companion to the source, but there is no strong evidence in favor of this hypothesis. In Section 5.3, we will impose the constraint on lens light: I_L > I_B .

As shown in Table 7, the source star lies 4.24 mag below the clump and is measured to have (V − I)_S,0 = 0.51 ± 0.09. This is unexpectedly blue, although it is within 1σ of a plausible value for a relatively metal-poor turnoff star. We attempt to check this measurement using KMTS data. However, these have too few magnified V-band points for a reliable measurement. We adopt the orientation that our normal error estimates adequately cover the measurement uncertainty. As discussed in Section 3.5, we obtain only a χ²(ρ) envelope function, which we will incorporate into the Bayesian analysis in Section 5.4. See Figure 10. Hence, the θ_ast determination in Table 7 does not lead to unambiguous estimates of θ_E and μ_rel. These can be fully investigated only in the context of the Bayesian analysis. For the moment, we express them in parametric form,

$$\begin{eqnarray}\begin{array}{rcl}{\theta }_{{\rm{E}}} & = & \displaystyle \frac{8.2\,\times \,{10}^{-4}}{\rho }\times (0.59\pm 0.07)\,\mathrm{mas};\\ {\mu }_{\mathrm{rel}} & = & \displaystyle \frac{8.2\,\times \,{10}^{-4}}{\rho }\times (6.1\pm 0.7)\,\mathrm{mas}\,{\mathrm{yr}}^{-1},\end{array}\end{eqnarray} \tag{ 19 }$$

where the prefactor has values ≃(1, 0.71, 0.57) at Δχ² = (1, 4, 9) of the envelope function. Thus, in contrast to many cases that lack a clear ρ measurement, the ρ constraint will play a significant role.

Zoom In Zoom Out Reset image size

As also discussed in Section 3.5, evaluating the angular radius of the second source in the 1L2S solution, θ_ast,2, is critically important to the kinematic argument against this solution. We present a new method for doing so, which is particularly adapted to mid- to late M dwarfs, for which it may be very difficult to make the color measurements that are needed for the traditional (Yoo et al. 2004) method. The first step is to note, from Tables 5 and 6, that this second source is 5.11 mag fainter than the source in the Local 1 2L1S model, which (from Table 7) is 4.22 mag fainter than the clump. That is, the second source is 9.33 ± 0.11 mag fainter than the clump, where the error is the quadrature sum of the errors in I_S,2 (Table 6) and I_cl (Table 7). We adopt I_cl,0 = 14.37 and M_I,cl = − 0.12.

We do not know, a priori, the exact distance of the source system along the line of sight. As noted above, the primary source appears to be a bulge turnoff star and so could in principle be anywhere in the bulge. We will consider below the full range of distances, but for the moment we adopt a fiducial distance modulus ${\mathrm{Dmod}}_{\mathrm{fid}}=14.37-(-0.12)+0.2\,=\,14.69$, i.e., 0.2 mag behind the clump centroid.

Next, we evaluate the second-source radius under the assumption that it lies exactly at this distance, and we initially ignore the error in its flux measurement. That is, we initially assume that it has an absolute magnitude M_I,2 = 9.33 +(−0.12) − 0.20 = 9.01. Using the mass–luminosity relations of Benedict et al. (2016) in V and K, together with the VIK color–color relations of Bessell & Brett (1988), we find that the mass of the putative second source would be M_S,2 =0.314 M_⊙. We then adopt the M-dwarf mass–radius relation (R/R_⊙) = (M/M_⊙) from Figure 7 of Parsons et al. (2018) and so obtain R₂ = 0.314 R_⊙ and thus θ_ast,2 = 0.169 μas.

We now take account of the fact that the source system could be at other distance moduli in the bulge. For example, if it were at 0.1 mag larger Dmod, then it would likewise be 0.1 mag more luminous, and so would have correspondingly larger mass (and radius), but the impact of this larger physical size on θ_ast,2 would be countered by the larger distance of the system. Applying the above arguments to arbitrary distances (within the bulge), we find ${{dM}}_{I}/d\mathrm{ln}M=-2.430$, and so (after a few steps)

$$\begin{eqnarray}&&{\theta }_{\mathrm{ast},2}=0.169\,\mu \mathrm{as}\times {10}^{-0.0213\,{\rm{\Delta }}\mathrm{Dmod}},\end{eqnarray} \tag{ 20 }$$

where ${\rm{\Delta }}\mathrm{Dmod}$ is the difference between the true distance modulus and the fiducial one adopted above. Stated alternatively, ${\theta }_{\mathrm{ast},2}\propto {D}_{S}^{0.046}$. Thus, for example, if we adopt ${\rm{\Delta }}\mathrm{Dmod}=0\pm 0.3$, then (still not taking account of the measurement error of σ(I_s,2) = 0.11) we find θ_ast,2 =0.169 ± 0.003 μas. The main error then comes from this measurement error, $\sigma (\mathrm{ln}{\theta }_{\mathrm{ast}})=0.11/2.43\,=\,0.045$. This leads to μ_rel = 1.16 ± 0.08 mas yr⁻¹, which we argued in Section 3.5.3 is highly unlikely.

Finally, the blended light lies on the foreground main sequence of the CMD in Figure 8. In principle, it might therefore be the lens or a companion to the lens. We therefore carefully investigate the offset between the magnified source and the baseline object Δ θ (N, E) = θ _base − θ _S . We make four measurements by applying two independent algorithms (pyDIA and pySIS) to two independent data sets (KMTC and KMTS). The best-fit values of the measurements are (in mas) Δ θ _KMTC,pyDIA = (+80, +72), Δ θ _KMTS,pyDIA = (+59, +24), Δ θ _KMTC,pySIS = (+61, +78), and Δ θ _KMTS,pySIS = (+106, + 142).

Before investigating the issue of measurement errors, we note that three of the four measurements lead to ∣Δ θ ∣ ≲ 0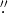1. The surface density of foreground-main-sequence stars that are brighter than the blend is just $39\,{\mathrm{arcmin}}^{-2}$, implying that the probability for a random field star to lie within 0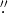1 is just p = 3.4 × 10⁻⁴. Thus, we must seriously consider the possibility that the apparent offset is due to measurement error.

The offset measurements have two sources of error: the error in the source position (derived from difference images), and the error in the baseline-object position (derived from point-spread function (PSF) photometry/astrometry of the baseline images). We expect the first of these to be small because the difference images are virtually free of systematic structures, apart from the magnified source. For example, in the two pySIS analyses, we find standard deviations from the six magnified images to be (again in mas) σ_KMTC = (21, 22) and σ_KMTS = (50, 25). The scatter is substantially smaller than the offsets, and it is plausible to treat these six measurements as independent, in which case the standard errors of the mean are substantially smaller yet.

However, it is substantially more difficult to estimate the errors in the DoPhot (Schechter et al. 1993) PSF-photometry measurement of the baseline-object position. While the baseline object appears isolated on the image, and the nearest neighbor in the star catalog is separated from the baseline object by 11, the astrometry of the baseline object could easily be corrupted by a faint field star. For example, an I = 20.75 star separated by 05 would not be separately resolved and would generate 01 error in the measured position. The surface density of such stars (without accounting for incompleteness at these faint magnitudes) is $608\,{\mathrm{arcmin}}^{-2}$, implying that the expected number within 05 is p = 13%. Therefore, it is quite plausible that the blended light is primarily due to the lens or a companion to the lens, although the evidence in favor of this scenario is certainly not definitive. We discuss the implications of this further in Section 5.4.

Because θ_E and π_E are both measured, it might appear that we could directly estimate the lens mass, M = θ_E/κ π_E, and distance, D_L = au(π_E θ_E + π_S ), where π_S ∼ 120 mas. However, because the two parallax solutions in Table 2 differ significantly, this procedure yields two different pairs of values: (M/M_⊙, D_L /kpc) = (0.18, 2.7) and (0.11, 1.7). Moreover, because the errors in π_E are not negligible, phase-space considerations will generally favor more distant lenses within each solution. Furthermore, they will also favor the u₀ > 0 solution owing to its smaller π_E. Hence, in order to take account of phase space and properly weight these two solutions, it is essential to conduct a Bayesian analysis. As constraints, we include t_E (Table 2), π _E (Equations (6) and (7)), and θ_E (Equations (15) and (16)). Note that the parallax error ellipses can also be expressed in equatorial coordinates, in which case the errors in the cardinal directions are given by Table 2 and the correlation coefficients are +0.53 and −0.73 for the u₀ > 0 and u₀ < 0 solutions, respectively. Formally, we also include the constraint on the lens flux I_L > I_B = 19.21, although as a practical matter it plays no role because the π_E and θ_E measurements already imply I_L ≳ 23.

The results shown in Table 8 confirm the naive reasoning given above. First, the median lens distances are larger than the naive estimates, while the 68% confidence intervals are skewed toward even larger distances. Second, because ${\theta }_{{\rm{E}}}\equiv \sqrt{\kappa M{\pi }_{\mathrm{rel}}}$ is better constrained than π_E, the "phase-space pressure" toward larger D_L (smaller π_rel) also pushes the host masses up relative to the naive estimates and likewise causes them to be asymmetric toward even higher values. Third, the "more populated" regions of phase space that are available to the u₀ > 0 solution, due to its smaller π_E, give it substantially higher Galactic-model weight, which more than compensates for its slightly worse χ².

Table 8. Physical Properties

Event					Relative Weights
Models	Physical Properties				Gal.Mod.	χ2
KB210712	Mhost (M☉)	Mplanet (M⊕)	D L (kpc)	a⊥ (au)
u0 > 0	${0.23}_{-0.07}^{+0.12}$	${39.90}_{-11.90}^{+20.60}$	${3.20}_{-0.68}^{+0.91}$	${2.29}_{-0.42}^{+0.64}$	1.000	0.374
u0 < 0	${0.14}_{-0.04}^{+0.06}$	${25.76}_{-7.11}^{+11.28}$	${2.08}_{-0.42}^{+0.65}$	${1.57}_{-0.26}^{+0.42}$	0.055	1.000
Adopted	${0.22}_{-0.07}^{+0.12}$	${38.19}_{-12.24}^{+20.52}$	${3.09}_{-0.75}^{+0.94}$	${2.22}_{-0.48}^{+0.66}$
KB210909	Mhost (M☉)	Mplanet (MJ)	D L (kpc)	a⊥ (au)
	${0.38}_{-0.20}^{+0.30}$	${1.26}_{-0.66}^{+1.01}$	${6.48}_{-1.38}^{+1.00}$	1.75 ± 0.42
KB212478	Mhost (M☉)	Mplanet (MJ)	D L (kpc)	a⊥ (au)
u0 > 0	${0.20}_{-0.05}^{+0.09}$	${0.91}_{-0.24}^{+0.42}$	2.86 ± 0.68	1.83 ± 0.30	0.866	0.992
u0 < 0	${0.20}_{-0.05}^{+0.10}$	${0.90}_{-0.24}^{+0.42}$	3.06 ± 0.71	1.87 ± 0.31	1.000	1.000
Adopted	${0.20}_{-0.05}^{+0.10}$	${0.90}_{-0.24}^{+0.42}$	2.97 ± 0.72	1.85 ± 0.31
KB211105	Mhost (M☉)	Mplanet (MJ)	D L (kpc)	a⊥ (au)
No IL constraint
Local 1	0.63 ± 0.33	1.30 ± 0.68	4.55 ± 1.67	${3.64}_{-1.19}^{+0.93}$	1.000	1.000
Local 2	0.63 ± 0.33	1.28 ± 0.67	4.48 ± 1.66	${2.82}_{-0.93}^{+0.72}$	0.975	0.129
IL > IB constraint
Local 1	0.61 ± 0.31	1.27 ± 0.65	4.62 ± 1.65	${3.60}_{-1.14}^{+0.89}$	0.946	1.000
Local 2	0.61 ± 0.32	1.24 ± 0.64	4.55 ± 1.64	${2.78}_{-0.89}^{+0.69}$	0.920	0.129
∣IL − IB ∣ < 0.2 const.
Local 1	${1.17}_{-0.31}^{+0.42}$	${2.43}_{-0.64}^{+0.88}$	4.14 ± 1.38	4.79 ± 0.87	0.023	1.000
Local 2	${1.17}_{-0.31}^{+0.42}$	${2.37}_{-0.63}^{+0.85}$	4.15 ± 1.38	3.70 ± 0.67	0.024	0.129
Adopted	0.63 ± 0.33	1.30 ± 0.68	4.54 ± 1.67	3.54 ± 1.06

Download table as:  ASCIITypeset image

The mass and distance distributions for the two solutions are shown in the top two rows of Figure 11.

Zoom In Zoom Out Reset image size

The planet is intermediate in mass between Neptune and Saturn and orbits a mid- to late M dwarf at about 3 kpc.

For this event, there is only one solution, for which there are two constraints: t_E = 16.06 ± 0.73 days (from Table 3) and θ_E = 0.362 ± 0.049 mas (from Equation (17)). The mass and distance distributions are presented in the third row of Figure 11. These show that the system most likely lies in the Galactic bulge.

The planet is of Jovian mass and orbits a mid-M dwarf at about 6.5 kpc.

For this event, there are four constraints (on t_E, π_E, I_L , and ρ). The constraints on t_E and π _E are given in Table 4, with the correlation coefficients for π _E being −0.07 and −0.11 for u₀ > 0 and u₀ < 0, respectively. As discussed in Section 4.3, the constraint on lens light is I_L > I_B = 18.15.

As discussed in Sections 3.4 and 4.3, the constraints on ρ are given by the χ²(ρ) envelope functions that are derived from the MCMC, which we implement as $\exp (-{\chi }^{2}(\rho )/2)$. See Figure 9.

Table 8 shows the results of the Bayesian analysis. The mass and distance are consistent at well under 1σ with the naive estimates given in Section 3.4.3 based on the relatively weak minima shown in Figure 9. The reason is that ρ is strongly constrained toward higher values by the MCMC, while substantially lower values are strongly disfavored by the Bayesian priors because they would imply very nearby lenses.

The mass and distance distributions are shown in Figure 11. Bulge lenses are virtually excluded. The planet is of Jovian mass and orbits a late M dwarf at about 3 kpc.

For this event there are two well-established constraints (on t_E and θ_E), as well as one potential constraint (on I_L ) that remains to be investigated. The first, from Table 5, is t_E = 35.0 ± 1.9 days (or t_E = 35.4 ± 1.9 days). The second, as discussed in Section 4.4, is implemented via a χ²(ρ) envelope function (Figure 10), together with an estimate for each simulated event with Einstein radius θ_E,i , of ρ_i = θ_ast/θ_E,i , with θ_ast given by Table 7.

The potential constraint on I_L comes from the limit I_L ≥ I_B,cal, where we calibrate the blend as I_B,cal = I_B − I_cl +I_cl,0 + A_I = 18.86, with I_B = 19.00 and I_cl − I_cl,0 = 2.88 coming from Table 7 and A_I = 2.75 coming from the KMT web page. The reason that this constraint is "potential" is that our investigation in Section 4.4 showed that the lens could be the origin of this blended light, in which case using it as a limit to exclude simulated events would bias the result toward lower masses. Thus, to check whether the "blend = lens" scenario is plausible within a Bayesian context, we first carry out the Bayesian analysis both with and without this constraint.

The results are given in Table 8 and illustrated in Figure 12. They show that the Bayesian estimates hardly change between the two cases. However, they also show that roughly 5% of the Galactic weight is eliminated by the flux constraint. This indicates that the "blend = lens" hypothesis is consistent with the Bayesian priors at about the 2σ level (without yet taking into consideration the low probability of finding a random field star ≲01 of the event).

Zoom In Zoom Out Reset image size

Therefore, we also conduct an additional Bayesian simulation under the constraint ∣I_L − I_B ∣ < 0.2. Note that the width of this interval is somewhat arbitrary: we just seek to distinguish simulated events that are roughly consistent with the "blend = lens" hypothesis from those that are not. The first point to note is that the host is a roughly solar-mass star at D_L ∼ 4 kpc. That is, it is substantially more massive than the unconstrained estimate, but roughly at the same distance. Second, at this distance, the lens system is located about 200 pc above the Galactic plane, and it therefore lies behind almost all the dust. Because [(V − I)_B − (V − I)_cl = − 0.46 (see Figure 8), this implies (V − I)_B,0 ≃ 0.60, which is a very plausible value for a solar-mass (or slightly more massive) star.

Thus, we find that the "blend = lens" scenario is consistent with all the available constraints. On the other hand, the hypothesis that the blend is a companion to the lens is also plausible. In this case, the ∼01 astrometric offset would be explained by the companion being roughly 400 au from the host (rather than corruption of the astrometry by a faint field star).

We conclude that the blend is very likely to be either the host or a companion to the host. These scenarios could easily be distinguished by high-resolution imaging by either AO on 8 m class telescopes or the HST. That is, a bright companion at ∼01 would easily be detected, which would verify the "blend = companion" scenario, while a contaminating random field star at several tenths of an arcsecond (verifying the "blend = lens" scenario) would be even more easily resolved. However, pending such a resolution, we advocate using the "no I constraint" Bayesian analysis, which we treat as "Adopted" in Table 8.

If future high-resolution imaging (which could be done immediately) confirms the "blend = lens" hypothesis, then KMT-2021-BLG-1105Lb would be one of the rare microlensing planets that could be further studied using the radial velocity (RV) technique. If we adopt M ≃ 1.1 M_⊙, which is consistent with both Table 8 and (V − I)_B,0 = 0.60, and we adopt π_rel ∼ 0.13 mas (consistent with Table 8), then ${\theta }_{{\rm{E}}}\,\equiv \sqrt{\kappa M{\pi }_{\mathrm{rel}}}\simeq 1.1\,\mathrm{mas}$ and a_⊥ ∼ 5.3 au (or 4.1 au). Considering that the semimajor axis is likely to be larger than the projected separation by a factor ∼1.5^1/2 = 1.22, the orbital periods for Locals 1 and 2 are likely of order 16 and 11 yr, respectively, with RV amplitudes of order $v\sin i\sim (25\,{\rm{m}}\,{{\rm{s}}}^{-1})\sin i$ and $\sim (30\,{\rm{m}}\,{{\rm{s}}}^{-1})\sin i$. Adopting A_I /E(V − I) ∼ 1.3 from Figure 6 of Nataf et al. (2013), we obtain V_B,cal = I_B,cal + (V − I)₀ +A_I /1.3 ≃ 21.6. Hence, assuming that future high-resolution imaging confirms that the blend is the lens, it will be feasible to carry out RV measurements of the requisite precision on this (I, V) ≃ (18.9, 21.6) star on 30 m class telescopes.