Observation of transverse spin Nernst magnetoresistance induced by thermal spin current in ferromagnet/non-magnet bilayers.

Kim DJ; Jeon CY; Choi JG; Lee JW; Surabhi S; Jeong JR; Lee KJ; Park BG

doi:10.1038/s41467-017-01493-5

Observation of transverse spin Nernst magnetoresistance induced by thermal spin current in ferromagnet/non-magnet bilayers.

Affiliations

1. Department of Materials Science and Engineering and KI for Nanocentury, KAIST, Daejeon, 34141, Korea.
Authors
Kim DJ¹
Jeon CY¹
Choi JG¹
Lee JW¹
Park BG¹
(5 authors)
2. Department of Materials Science and Engineering, Graduate School of Energy Science Technology, Chungnam National University, Daejeon, 34134, Korea.
Authors
Surabhi S²
Jeong JR²
(2 authors)
3. Department of Materials Science and Engineering, Korea University, Seoul, 02841, Korea.
Authors
Lee KJ³
(1 author)

ORCIDs linked to this article

Show all (6)

Nature Communications, 09 Nov 2017, 8(1):1400
https://doi.org/10.1038/s41467-017-01493-5 PMID: 29123123 PMCID: PMC5680200

This article is in the Europe PMC Open access subset. Refer to the copyright information in the article for licensing details.

Free full text in Europe PMC

This article has been corrected. See Nat Commun. 2018 Jan 5;9(1):138.

Abstract

Electric generation of spin current via spin Hall effect is of great interest as it allows an efficient manipulation of magnetization in spintronic devices. Theoretically, pure spin current can be also created by a temperature gradient, which is known as spin Nernst effect. Here, we report spin Nernst effect-induced transverse magnetoresistance in ferromagnet/non-magnetic heavy metal bilayers. We observe that the magnitude of transverse magnetoresistance in the bilayers is significantly modified by heavy metal and its thickness. This strong dependence of transverse magnetoresistance on heavy metal evidences the generation of thermally induced pure spin current in heavy metal. Our analysis shows that spin Nernst angles of W and Pt have the opposite sign to their spin Hall angles. Moreover, our estimate implies that the magnitude of spin Nernst angle would be comparable to that of spin Hall angle, suggesting an efficient generation of spin current by the spin Nernst effect.

Free full text

Nat Commun. 2017; 8: 1400.

Published online 2017 Nov 9. https://doi.org/10.1038/s41467-017-01493-5

PMCID: PMC5680200

PMID: 29123123

Observation of transverse spin Nernst magnetoresistance induced by thermal spin current in ferromagnet/non-magnet bilayers

Dong-Jun Kim,¹ Chul-Yeon Jeon,¹ Jong-Guk Choi,¹ Jae Wook Lee,¹ Srivathsava Surabhi,² Jong-Ryul Jeong,² Kyung-Jin Lee,^3,⁴ and Byong-Guk Park¹

Author information Article notes Copyright and License information Disclaimer

This article has been corrected. See Nat Commun. 2018 January 5; 9: 138.

This article has been cited by other articles in PMC.

Go to:

Associated Data

Supplementary Materials: Supplementary Information
41467_2017_1493_MOESM1_ESM.pdf (1.7M)
Peer Review File
41467_2017_1493_MOESM2_ESM.pdf (1009K)

Data Availability Statement: Authors can confirm that all relevant data are included in the paper and/or its supplementary information files and data are available on request.

Go to:

Abstract

Go to:

Introduction

A central theme of spintronics field is the electrical generation of a spin current as the spin current allows for an efficient magnetization switching and a high speed domain wall motion in magnetic nanostructures^¹–⁶. In ferromagnet (FM)/non-magnetic heavy metal (HM) bilayers, a longitudinal charge current creates a transverse spin current via spin Hall effect (SHE)^{⁷, ⁸}. The spin current induces spin accumulation at the FM/HM interface, which exerts a torque on the FM and controls the magnetization direction^{¹, ²}. On the other hand, the spin current is partially reflected from the FM/HM interface depending on its spin orientation with respect to the magnetization direction of the FM layer. This reflected spin current is then converted to a charge current via inverse SHE (ISHE), resulting in the variation of the longitudinal resistance of the FM/HM bilayers, i.e., spin Hall magnetoresistance (SMR)^⁹–¹¹. As the SMR originates from the SHE-induced spin current and the ISHE of the reflected spin current, its magnitude depends on the square of the spin Hall angle (θ _SH), charge-to-spin conversion efficiency.

Spin current is also generated by a temperature gradient, for instance, the spin (-dependent) Seebeck effect in FM/non-magnetic bilayer structures where thermally induced spin current is injected from the FM into the non-magnetic layer^¹²–¹⁷. Theories have predicted that a pure spin current is thermally generated in non-magnetic materials by their spin–orbit coupling effects^¹⁸–²¹, a thermal analog to the SHE, i.e., spin Nernst effect (SNE) (Fig. 1a). However, there has been no experimental observation yet on the thermally induced pure spin current or SNE.

Fig. 1

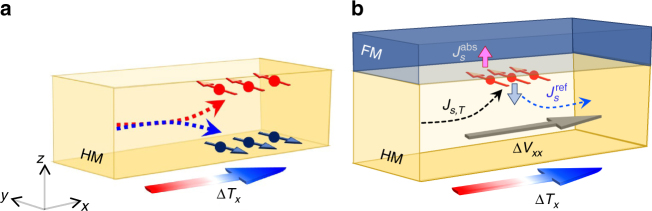
Schematics for spin Nernst effect and spin Nernst magnetoresistance. a Spin Nernst effect (SNE), where the temperature gradient in x-direction generates a spin current in z-direction with the spin orientation in y-direction. b Spin Nernst magnetoresistance (SNMR) in FM/HM bilayer where a spin current induced in HM by a temperature gradient in x-direction (J _s,T) partially reflected at the FM/HM interface depending on its spin orientation with respect to the magnetization direction of the FM layer, resulting in the modification of the longitudinal (ΔV _xx) and transverse (ΔV _xy) thermoelectric voltages of the bilayer. $J_{s}^{abs}$ ( $J_{s}^{ref}$ ) is the absorbed (reflected) spin current at the FM/HM interface

In this work, we demonstrate the SNE by investigating the Hall resistance variation of the FM/HM bilayers under a temperature gradient. Similar to the SMR originating from combined effects of SHE (charge-to-spin conversion) and ISHE (spin-to-charge conversion), the SNE could also cause a resistance variation of the bilayer. This thermally induced magnetoresistance in a FM/HM bilayer, which can be called spin Nernst magnetoresistance (SNMR), originates from combined effects of two processes: (i) SNE-induced spin current in HM, of which efficiency is described by spin Nernst angle (θ _SN), heat-to-spin conversion efficiency, and (ii) subsequent reflection of a spin current at the FM/HM interface and conversion to a charge current via ISHE, of which efficiency is described by θ _SH (Fig. 1b). As a result, the magnitude of SNMR is determined by the product of θ _SN and θ _SH. In analogous to a modification of the planar Hall effect signal (i.e., transverse SMR) by the SHE^{¹¹, ²²}, the SNE modifies the planar Nernst effect signal (i.e., transverse SNMR). Therefore, a systematic investigation of transverse SNMR in FM/HM bilayers allows us to identify the SNE, which we have done in this work. We find that θ _SN has a comparable magnitude to θ _SH for W and Pt, suggesting that the SNE can efficiently create a spin current as much as the SHE can do.

Go to:

Results

Spin Nernst magnetoresistance model

The SNMR in a FM/HM bilayer can be described by replacing SHE-induced spin current with thermal pure spin current, $J_{s, T} = - θ_{SN} σ_{HM} S_{HM} \frac{\partial T}{\partial x}$ , in the SMR model^⁹–¹¹, where σ _HM and S _HM are the electrical conductivity and the Seebeck coefficient of the HM, respectively. The longitudinal $(Δ V_{x x})$ and transverse $(Δ V_{x y})$ thermoelectric voltages caused by the longitudinal and transverse SNMRs are respectively expressed as,

\frac{Δ V_{x x}}{L_{V}} = - [S_{0} + Δ S_{1} + Δ S_{2} (1 - m_{y}^{2})] \frac{Δ T_{x}}{L_{T}},

\frac{Δ V_{x y}}{L_{V}} = - [Δ S_{2} m_{x} m_{y} + Δ S_{3} m_{z}] \frac{Δ T_{x}}{L_{T}},

where L _T(V) is the effective length for temperature gradient (thermal voltage generation), m _x , m _y, and m _z are the x, y, and z component of the magnetization, respectively, $Δ T_{x}$ is the temperature difference along the x-axis induced from localized thermal excitation, and

Δ S_{1} \equiv - χ_{HM} θ_{SH} θ_{SN} S_{HM} \frac{2 λ}{d_{HM}} tanh (\frac{d_{HM}}{2 λ}),

Δ S_{2} \equiv χ_{HM} θ_{SH} θ_{SN} S_{HM} Re \frac{λ}{d_{HM}} \frac{2 λ G \tan h^{2} (\frac{d_{HM}}{2 λ})}{σ_{HM} + 2 λ G \coth (\frac{d_{HM}}{λ})},

Δ S_{3} \equiv - χ_{HM} θ_{SH} θ_{SN} S_{HM} Im \frac{λ}{d_{HM}} \frac{2 λ G \tan h^{2} (\frac{d_{HM}}{2 λ})}{σ_{HM} + 2 λ G \coth (\frac{d_{HM}}{λ})},

and S ₀ is the ordinary Seebeck coefficient in the bilayer structure. Here, ΔS ₁, ΔS ₂, and ΔS ₃ are additional Seebeck coefficients induced by SNE, where d _HM and λ are the thickness and spin diffusion length of the HM, respectively, and G is the spin mixing conductance of the FM/HM interface. Note that SNE in FM layer and inverse SNE in HM are ignored and the shunting effect of FM layer is taken into consideration using a geometric factor, χ _HM=((σ _HM d _HM)/(σ _HM d _HM+σ _FM d _FM)), where σ _FM and d _FM are the electrical conductivity and thickness of the FM layer, respectively. The ΔV _xx depends on the magnetization direction relative to the spin orientation (y) of SNE-induced spin current, and it is thus proportional to $m_{y}^{2}$ while its magnitude is determined by the ΔS ₂. As our samples have in-plane magnetization (m _z≈0), the ΔV _xy ( [proportional, variant] m _x m _y) has the same magnitude (ΔS ₂) as that of the ΔV _xx, so that the investigation of ΔV _xy corresponding to the transverse SNMR (or planar Nernst effect (PNE) signal) allows us to explore the SNE. Note that the sign of the SNMR is determined by the sign of the product of θ _SH, θ _SN, and S _HM of the HM, which is distinct from the fact that the sign of the SMR is independent of the sign of θ _SH.

Transverse spin Nernst magnetoresistance in W/CoFeB

We first examine the transverse SNMR in W(3nm)/Co₃₂Fe₄₈B₂₀(CoFeB, 2nm) sample, in which a thermal gradient is generated by a focused laser (55mW) of ~5μm diameter. Figure 2a schematically illustrates the experiment setup where thermoelectric Hall voltage is measured as a function of in-plane magnetic field angle θ with respect to the x-axis under a temperature gradient. The magnetization is aligned parallel to the applied magnetic field of 100mT. Depending on the laser position in the sample structure, a vertical (ΔT _z) and/or lateral (ΔT _x) temperature differences in the sample are created accordingly (Supplementary Note ¹). Upon illumination with a laser spot at the center of the sample (Fig. 2a, b), generating only ΔT _z while ΔT _x cancels out, the thermoelectric signal of W/CoFeB sample shows a clear cosθ dependence ( [proportional, variant] m _x); the largest value (zero) for θ=0 (θ=±90), where the magnetization is aligned to the x-axis (y-axis). This reveals that the signal originates from the longitudinal spin Seebeck effect and anomalous Nernst effect^²³. On the other hand, as the laser spot moves toward the edge of the sample, the laser illumination generates non-zero ΔT _x and as a result, an additional angle-dependent thermoelectric Hall signal appears, which is proportional to m _x m _y or sin2θ. The sin2θ signal reverses its sign upon the change in the direction of ΔT _x while the cosθ signal remains the same sign, which is demonstrated in the Fig. 2c where two angle-dependent signals are decomposed. The sin2θ signal eventually dominates the total signal when the laser spot moves further away, where ΔT _z induced in the sample is negligible. (see the Fig. 2d), confirming that it originates from ΔT _x. Note that the thermoelectric signals are almost independent of the magnetic field when it is large enough to saturate the magnetization (Supplementary Note ²), and the laser position along the y-axis (Supplementary Note ³). The latter is due to the local excitation by the laser heating (diameter ~5μm) in the elongated sample structure: 10μm×1mm in which the ΔT _y between the two ends of the sample is not significantly generated by the laser illumination.

Fig. 2

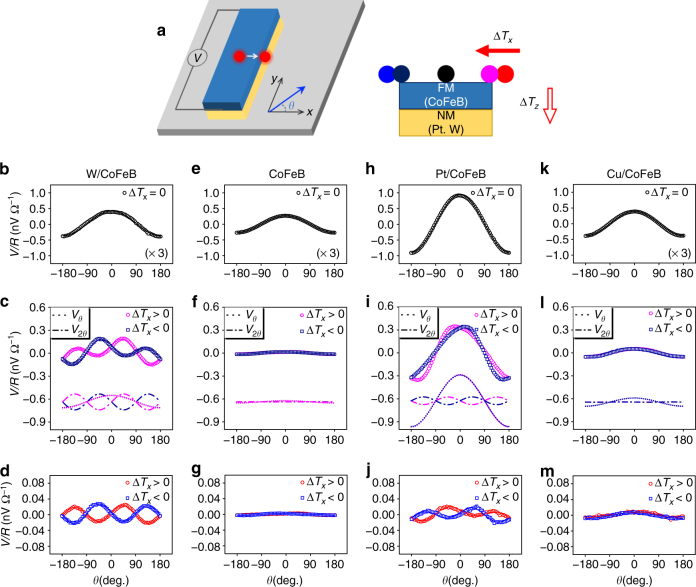
Transverse spin Nernst magnetoresistance originating from SNE in various layer structures. a Schematics of measurement under different laser position on bar-shaped structure. The x–z plane view indicates the laser positions along the x direction. Each color of circle represents the laser position. b–m Thermoelectric Hall signals for W(3nm)/CoFeB(2nm) (b–d), CoFeB(2nm) (e–g), Pt(3nm)/CoFeB(2nm) (h–j), and Cu(3nm)/CoFeB(2nm) structures (k–m) for different laser locations, at the center (x~0μm, b, e, h, k), edge (x~5μm, c, f, i, l), and outside of the structure (x~10μm, d, g, j, m) for each sample, which are normalized by sample resistance. Dotted and dash-dotted lines (c, f, i, l) show the decomposition of two angle-dependent signals of cosθ and sin2θ. The symbol color denotes the laser position as illustrated in schematics of Fig. 2a

The signal induced by ΔT _x ( [proportional, variant] m _x m _y) has the same symmetry as PNE, but the magnitude is noticeably large in the W/CoFeB sample as compared to that in the control sample of a single CoFeB (2nm) layer (see the decomposed dotted lines in the Fig. 2f). As the ΔT _x in a single CoFeB is comparable to that of W/CoFeB layer (Supplementary Note ¹), the large enhancement in PNE indicates that there is a significant contribution from the W layer to the sin2θ thermoelectric signal, which we attribute to the consequence of transverse SNMR caused by a thermal generation of pure spin current in W and subsequent reflection of the spin current at the W/CoFeB interface depending on the magnetization direction. Note that the enhanced PNE signal is due to the transverse component of the SNMR as the planar Hall effect in the same W/CoFeB sample is strongly modified by the transverse SMR (Supplementary Note ⁴). As the transverse SNMR depends on SNE-induced spin current and its conversion into charge voltage via the ISHE, the sign of the transverse SNMR and equivalently the sign of the PNE corresponding to the SNE are determined by the product of the θ _SH and θ _SN. For W, it is known as θ _SH<0^¹¹ ^, ^²² ^, ^²⁴ and S _HM>0^²⁵, thus the positive transverse SNMR for ΔT _x>0 indicates that a positive θ _SN for W, which is the opposite sign to its θ _SH. We note that this sign difference is not impossible because θ _SH is determined by the density of states at the Fermi energy while θ _SN is determined by the energy derivative of density of states^²⁰.

Material dependence of spin Nernst magnetoresistance

We also investigate the transverse SNMR for different non-magnetic materials such as Pt and Cu. Note that Pt has a positive θ _SH ^{⁹, ¹², ²⁴, ²⁶}, the opposite sign to that of W, while Cu has a negligible θ _SH ^{⁹, ²⁴}. Figure 2h, k show that under the central heating, both samples exhibit cosθ angular dependence as the W/CoFeB or CoFeB sample does (see the Fig. 2b, e). When a sizable ΔT _x is applied, on the other hand, the sin2θ thermoelectric signal exhibits a strong material dependence; an opposite sign for the Pt/CoFeB sample (Fig. 2i, j) and negligibly small for the Cu/CoFeB sample (Fig. 2l, m) as compared to that of the W/CoFeB sample. As the same thickness of CoFeB is used and a similar ΔT _x is induced for all samples (Supplementary Note ¹), these results again confirm that the sin2θ thermoelectric signal is dominated by the SNE-induced spin current in HM through its spin–orbit coupling effects.

Estimation of spin Nernst angle

We next estimate the heat-to-spin conversion coefficient θ _SN using the HM layer thickness dependence of the transverse SNMR in HM/CoFeB samples. We note that the accuracy of this estimation substantially depends on the accuracy of ΔT _x and ΔT _z. As it is hard to experimentally determine ΔT _x and ΔT _z, we estimate the temperature distribution of the sample under the laser illumination by solving the heat transfer module of the COMSOL software (Supplementary Note ¹) and a control sample (Supplementary Fig. ⁵). As a result, we do not argue that our estimation of θ _SN is quantitatively accurate, but we believe that it is still meaningful to estimate θ _SN even approximately.

We performed the same measurement shown in Fig. 2 while varying the laser positions from the center to the edge of the samples, and then separated the cosθ and sin2θ components (V _θ, V _2θ). The latter corresponds to the transverse SNMR which is summarized in Fig. 3a, b for W/CoFeB and Pt/CoFeB samples, respectively (see Supplementary Note ⁵ for more details). The V _2θ shows the peak values when the laser is located at the edge of the sample (x~5μm), where ΔT _x is maximized. Figure 3c show the V _2θ of W/CoFeB samples for the edge illumination as a function of W thickness, demonstrating that the V _2θ becomes the largest at 4nm of W and decreases with a further increase in W thickness. This is the same trend as the W thickness dependence of the SMR in similar W/CoFeB structures (ref. ^²² and Supplementary Note ⁴), indicating that the spin transport in W dominantly governs the transverse SNMR of our samples. A similar thickness dependence of the transverse SNMR is also observed for the Pt/CoFeB samples, which is shown in Fig. 3d. In order to estimate θ _SN, we fit the thickness dependence of the transverse SNMR to Eq. (²) using material parameters (Table 1) and the calculated ΔT _x that is obtained to be ~24K for W/CoFeB and Pt/CoFeB samples, and ~17K for CoFeB sample when the laser of 55mW illuminates at the edge of the sample (Supplementary Fig. ⁴). Note that the variation of resistivity in W with its thickness has been taken into account (Supplementary Note ⁶). From the fitting, we obtained θ _SN values of 0.22–0.42 for W and −0.12 to −0.24 for Pt, and λ values of (2.0±0.1)nm for W, and (1.0±0.1)nm for Pt. The purple bands in Fig. 3c, d indicate error ranges which possibly originates from uncertainties (±30%) of the literature values of S _HM, G, and θ _SH. Note that the Seebeck coefficient of Pt is negative (S _HM<0)^²⁵, which is an opposite sign to that of W. This fitting result demonstrates that θ _SN has a comparable magnitude to θ _SH but has an opposite sign to θ _SH for both W and Pt (Table 1). The comparable magnitude between θ _SN and θ _SH implies that the SNE in HM layer can create a spin current as much as the SHE can if a thermal gradient is efficiently generated.

Fig. 3

Thickness dependence of transverse SNMR in W/CoFeB and Pt/CoFeB structures. a, b Laser-position-dependent thermoelectric signal V _2θ for W/CoFeB structure (a) and Pt/CoFeB structure (b) with different HM thicknesses ranging from 1 to 5nm. Black arrow represents position of edge illumination. c, d HM thickness dependence of the V _2θ for edge illumination for W/CoFeB structure (c) and Pt/CoFeB structure (d). The white circles represent experimental data and solid lines represent best fitted curves, while purple band indicates error ranges of extracted values, which originated from uncertainties of S _HM, G, and θ _SH. Error bars represent the range of the measured values, resulting from laser-position uncertainty

Table 1

Parameters for analysis of transverse spin Nernst magnetoresistance

	1/σ _HM (μΩcm)	1/σ _FM (μΩcm)	θ _SH	G (Ω⁻¹m ⁻²)	S _HM (μVK⁻¹)	λ (nm)	θ _SN
W/CoFeB	35–125	320	−0.21^{²², ²⁴}	0.5–5×10¹⁵ ^{¹¹, ²²}	10^²⁵	2.0±0.1	0.22 to 0.42
Pt/CoFeB	30	320	0.10^{²⁴, ²⁶}	0.5–5×10¹⁵ ^{¹⁰, ²⁶}	−10^²⁵	1.0±0.1	−0.12 to −0.24

Go to:

Discussion

We demonstrate the transverse SNMR in HM/FM bilayers which signifies an efficient thermal generation of spin current by SNE. Our estimation of the heat-to-spin conversion efficiency θ _SN of W or Pt implies that the magnitude of θ _SN could be comparable to that of the charge-to-spin conversion efficiency θ _SH. This suggests that the SNE-induced spin current could create a considerable spin torque to adjacent FM layer, or thermal spin–orbit torques that can manipulate the magnetization direction of the FM as electrical spin–orbit torques do. Moreover, thermal spin–orbit torque can be combined with electrical spin–orbit torque by applying both a charge current and a thermal gradient to bilayers, which allows for the reduction in the critical current for magnetization switching. These results open up an alternative way to generate the spin current and/or to control the magnetization direction in spintronic devices.

We would like to note that while we were preparing the manuscript, we became aware that similar work has been done by other groups^{²⁷, ²⁸}.

Go to:

Methods

Sample preparation

All samples of W/Co₃₂Fe₄₈B₂₀(CoFeB), Pt/CoFeB, and CoFeB were prepared by magnetron sputtering on thermally oxidized Si substrates with a base pressure of less than 4.0×10⁻⁶Pa (3.0×10⁻⁸Torr) at room temperature. All samples were covered by MgO (1nm)/Ta (1nm) capping layer to prevent oxidation. The bar-shaped structures of 10μm×1 mm dimension for SNMR measurement are patterned using photolithography and Ar ion milling. The resistivities are measured to be 320×10⁻⁸ Ωm⁻¹ for CoFeB, 30×10⁻⁸ Ωm⁻¹ for Pt, while that of W is 112×10⁻⁸ Ωm⁻¹ when W is thinner than 4nm and it gradually decreases with its thickness greater than 4nm.

Transverse spin Nernst magnetoresistance measurements

The thermoelectric Hall voltage along the y-axis was measured under the temperature gradients ( [nabla] T _x, [nabla] T _z)in the sample, which were generated by laser illumination of 55mW, while rotating a magnetic field of 100mT in the x–y plane which is larger than in-plane anisotropy field of CoFeB layer. The measurements were repeated at each laser position varying from center to edge of the sample, which was monitored by its reflectance of the laser. All measurements were carried out at room temperature and each measurement was repeated more than three times; data are reproducible.

Data availability

Authors can confirm that all relevant data are included in the paper and/or its supplementary information files and data are available on request.

Go to:

Electronic supplementary material

Supplementary Information^{(1.7M, pdf)}

Peer Review File^{(1009K, pdf)}

Go to:

Acknowledgements

This work was supported by the National Research Foundation of Korea (NRF-2015M3D1A1070465, 2014R1A2A1A11051344, and 2016R1A2B4012847 and 2017R1A2B2006119) and the DGIST R&D Program of the Ministry of Science, ICT and Future Planning (17-BT-02).

Go to:

Author contributions

B.-G.P. planned and supervised the study. D.-J.K. and C.-Y.J. fabricated devices. D.-J.K., C.-Y.J., J.-G.C. and J.W.L. performed spin thermoelectric and transport measurement. S.S. and J.-R.J. simulated the temperature distribution. D.-J.K., K.-J.L. and B.-G.P. analyzed the results and wrote the manuscript.

Go to:

Notes

Go to:

Competing interests

The authors declare no competing financial interests.

Go to:

Footnotes

A correction to this article is available online at https://doi.org/10.1038/s41467-017-02303-8.

Electronic supplementary material

Supplementary Information accompanies this paper at 10.1038/s41467-017-01493-5.

Change history: A correction to this article has been published and is linked from the HTML version of this paper.

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Go to:

References

1. Miron IM, et al. Perpendicular switching of a single ferromagnetic layer induced by in-plane current injection. Nature. 2011;476:189–193. 10.1038/nature10309. [Abstract] [CrossRef] [Google Scholar]

2. Liu L, et al. Spin-torque switching with the giant spin Hall effect of tantalum. Science. 2012;336:555–558. 10.1126/science.1218197. [Abstract] [CrossRef] [Google Scholar]

3. Ryu K-S, Thomas L, Yang S-H, Parkin S. Chiral spin torque at magnetic domain walls. Nat. Nanotech. 2013;8:527–533. 10.1038/nnano.2013.102. [Abstract] [CrossRef] [Google Scholar]

4. Emori S, Bauer U, Ahn S-M, Martinez E, Beach GSD. Current-driven dynamics of chiral ferromagnetic domain walls. Nat. Mater. 2013;12:611–616. 10.1038/nmat3675. [Abstract] [CrossRef] [Google Scholar]

5. Fukami S, Anekawa T, Zhang C, Ohno H. A spin-orbit torque switching scheme with collinear magnetic easy axis and current configuration. Nat. Nanotech. 2016;11:621–625. 10.1038/nnano.2016.29. [Abstract] [CrossRef] [Google Scholar]

6. Oh Y-W, et al. Field-free switching of perpendicular magnetization through spin-orbit torque in antiferromagnet/ferromagnet/oxide structures. Nat. Nanotech. 2016;11:878–884. 10.1038/nnano.2016.109. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]

7. Dyakonov MI, Perel VI. Current-induced spin orientation of electrons in semiconductors. Phys. Lett. A. 1971;35A:459–460. 10.1016/0375-9601(71)90196-4. [CrossRef] [Google Scholar]

8. Sinova J, Valenzuela SO, Wunderlich J, Back CH, Jungwirth T. Spin Hall effects. Rev. Mod. Phys. 2015;87:1213–1259. 10.1103/RevModPhys.87.1213. [CrossRef] [Google Scholar]

9. Nakayama H, et al. Spin Hall magnetoresistance induced by a nonequilibrium proximity effect. Phys. Rev. Lett. 2013;110:206601. 10.1103/PhysRevLett.110.206601. [Abstract] [CrossRef] [Google Scholar]

10. Chen Y-T, et al. Theory of spin Hall magnetoresistance. Phys. Rev. B. 2013;87:144411. 10.1103/PhysRevB.87.144411. [CrossRef] [Google Scholar]

11. Kim J, Sheng P, Takahashi S, Mitani S, Hayashi M. Spin Hall magnetoresistance in metallic bilayers. Phys. Rev. Lett. 2016;116:097201. 10.1103/PhysRevLett.116.097201. [Abstract] [CrossRef] [Google Scholar]

12. Uchida K, et al. Observation of the spin Seebeck effect. Nature. 2008;455:778–781. 10.1038/nature07321. [Abstract] [CrossRef] [Google Scholar]

13. Bauer GEW, Saitoh E, van Wees BJ. Spin caloritronics. Nat. Mater. 2012;11:391–399. 10.1038/nmat3301. [Abstract] [CrossRef] [Google Scholar]

14. Slachter A, Bakker FL, Adam JP, van Wees BJ. Thermally driven spin injection from a ferromagnet into a non-magnetic metal. Nat. Phys. 2010;6:879–882. 10.1038/nphys1767. [CrossRef] [Google Scholar]

15. Le Breton J-C, Sharma S, Saito H, Yuasa S, Jansen R. Thermal spin current from a ferromagnet to silicon by Seebeck spin tunneling. Nature. 2011;475:82–85. 10.1038/nature10224. [Abstract] [CrossRef] [Google Scholar]

16. Choi GM, Moon CH, Min BC, Lee KJ, Cahill DG. Thermal spin-transfer torque driven by the spin-dependent Seebeck effect in metallic spin-valves. Nat. Phys. 2015;11:576–581. 10.1038/nphys3355. [CrossRef] [Google Scholar]

17. Qu D, Huang SY, Hu J, Wu R, Chien CL. Intrinsic spin Seebeck effect in Au/YIG. Phys. Rev. Lett. 2013;110:067206. 10.1103/PhysRevLett.110.067206. [Abstract] [CrossRef] [Google Scholar]

18. Cheng S, Xing Y, Sun Q, Xie XC. Spin Nernst effect and Nernst effect in two-dimensional electron systems. Phys. Rev. B. 2008;78:045302. 10.1103/PhysRevB.78.045302. [CrossRef] [Google Scholar]

19. Liu X, Xie X. Spin Nernst effect in the absence of a magnetic field. Solid State Commun. 2010;150:471–474. 10.1016/j.ssc.2009.12.017. [CrossRef] [Google Scholar]

20. Tauber K, Gradhand M, Fedorov DV, Mertig I. Extrinsic spin Nernst effect from first principles. Phys. Rev. Lett. 2012;109:026601. 10.1103/PhysRevLett.109.026601. [Abstract] [CrossRef] [Google Scholar]

21. Wimmer S, Ködderitzsch D, Chadova K, Ebert H. First-principles linear response description of the spin Nernst effect. Phys. Rev. B. 2013;88:201108(R). 10.1103/PhysRevB.88.201108. [CrossRef] [Google Scholar]

22. Cho S, Beak S-hC, Jo Y, Park B-G. Large spin Hall magnetoresistance and its correlation to the spin-orbit torque in W/CoFeB/MgO structures. Sci. Rep. 2015;5:14668. 10.1038/srep14668. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]

23. Lee K-D, et al. Thermoelectric signal enhancement by reconciling the spin Seebeck and anomalous Nernst effects in ferromagnet/non-magnet multilayers. Sci. Rep. 2015;5:10249. 10.1038/srep10249. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]

24. Wang HL, et al. Scaling of spin Hall angle in 3d, 4d, and 5d metals from Y₃Fe₅O₁₂/metal spin pumping. Phys. Rev. Lett. 2014;112:197201. 10.1103/PhysRevLett.112.197201. [Abstract] [CrossRef] [Google Scholar]

25. Abadlia L, Gasser F, Khalouk K, Mayoufi M, Gasser JG. New experimental methodology, setup and LabView program for accurate absolute thermoelectric power and electrical resistivity measurements between 25 and 1600 K: Application to pure copper, platinum, tungsten, and nickel at very high temperatures. Rev. Sci. Instrum. 2014;85:095121. 10.1063/1.4896046. [Abstract] [CrossRef] [Google Scholar]

26. Rezende SM, et al. Enhanced spin pumping damping in yttrium iron garnet/Pt bilayers. Appl. Phys. Lett. 2013;102:012402. 10.1063/1.4773993. [CrossRef] [Google Scholar]

27. Meyer S, et al. Observation of the spin Nernst effect. Nat. Mater. 2017;16:977–981. 10.1038/nmat4964. [Abstract] [CrossRef] [Google Scholar]

28. Sheng, P., Sakuraba, Y., Takahashi, S., Mitani, S. & Hayashi, M. Signatures of the spin Nernst effect in Tungsten. Preprint at http://arxiv.org/abs/1607.06594 (2016). [Europe PMC free article] [Abstract]

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

Full text links

Read article at publisher's site: https://doi.org/10.1038/s41467-017-01493-5

Read article for free, from open access legal sources, via Unpaywall: https://www.nature.com/articles/s41467-017-01493-5.pdf

Citations & impact

Impact metrics

Citations

Jump to Citations

Citations of article over time

Alternative metrics

Altmetric item for https://www.altmetric.com/details/25170539

Altmetric
Discover the attention surrounding your research
https://www.altmetric.com/details/25170539

Article citations

Thermally generated spin current in the topological insulator Bi₂Se₃.
Jain R, Stanley M, Bose A, Richardella AR, Zhang XS, Pillsbury T, Muller DA, Samarth N, Ralph DC
Sci Adv, 9(50):eadi4540, 13 Dec 2023
Cited by: 1 article | PMID: 38091392 | PMCID: PMC10848729
This article is in the Europe PMC Open access subset. Refer to the copyright information in the article for licensing details.
Free full text in Europe PMC
Transport phenomena in spin caloritronics.
Uchida KI
Proc Jpn Acad Ser B Phys Biol Sci, 97(2):69-88, 01 Jan 2021
Cited by: 5 articles | PMID: 33563879 | PMCID: PMC7897901
Review
This article is in the Europe PMC Open access subset. Refer to the copyright information in the article for licensing details.
Free full text in Europe PMC
Precise Determination of the Temperature Gradients in Laser-irradiated Ultrathin Magnetic Layers for the Analysis of Thermal Spin Current.
Surabhi S, Kim DJ, Cao Van P, Dong Quoc V, Kim JM, Lee SW, Kuchi R, Lee JW, Yoon SG, Choi J, Park BG, Jeong JR
Sci Rep, 8(1):11337, 27 Jul 2018
Cited by: 1 article | PMID: 30054593 | PMCID: PMC6063919
This article is in the Europe PMC Open access subset. Refer to the copyright information in the article for licensing details.
Free full text in Europe PMC

Data

Data behind the article

This data has been text mined from the article, or deposited into data resources.

BioStudies: supplemental material and supporting data

http://www.ebi.ac.uk/biostudies/studies/S-EPMC5680200?xr=true

Search life-sciences literature (45,104,206 articles, preprints and more)

Observation of transverse spin Nernst magnetoresistance induced by thermal spin current in ferromagnet/non-magnet bilayers.

Author information

Affiliations

Authors

Authors

Authors

ORCIDs linked to this article

Abstract

Free full text

Observation of transverse spin Nernst magnetoresistance induced by thermal spin current in ferromagnet/non-magnet bilayers

Dong-Jun Kim

Chul-Yeon Jeon

Jong-Guk Choi

Jae Wook Lee

Srivathsava Surabhi

Jong-Ryul Jeong

Kyung-Jin Lee

Byong-Guk Park

Associated Data

Abstract

Introduction

Results

Spin Nernst magnetoresistance model

Transverse spin Nernst magnetoresistance in W/CoFeB

Material dependence of spin Nernst magnetoresistance

Estimation of spin Nernst angle

Table 1

Discussion

Methods

Sample preparation

Transverse spin Nernst magnetoresistance measurements

Data availability

Electronic supplementary material

Acknowledgements

Author contributions

Notes

Competing interests

Footnotes

References

Full text links

Citations & impact

Impact metrics

Citations of article over time

Alternative metrics

Article citations

Thermally generated spin current in the topological insulator Bi2Se3.

Transport phenomena in spin caloritronics.

Precise Determination of the Temperature Gradients in Laser-irradiated Ultrathin Magnetic Layers for the Analysis of Thermal Spin Current.

Data

Data behind the article

BioStudies: supplemental material and supporting data

Similar Articles

The spin Nernst effect in tungsten.

Large spin Hall magnetoresistance and its correlation to the spin-orbit torque in W/CoFeB/MgO structures.

Publisher Correction: Observation of transverse spin Nernst magnetoresistance induced by thermal spin current in ferromagnet/non-magnet bilayers.

Thermally generated spin current in the topological insulator Bi2Se3.

Partnerships & funding

Thermally generated spin current in the topological insulator Bi₂Se₃.

Thermally generated spin current in the topological insulator Bi₂Se₃.