This site is supported by donations to The OEIS Foundation.
User:Rémy Sigrist
Interested in mathematics and computing from a young age.
Wave curve
I came across this fractal while playing with Koch curve.
- To build a Koch curve: iteratively substitute to a segment AE a broken line ABCDE where A, B, D, E are aligned and equally spaced and BCD forms an equilateral triangle.
- To build this curve: apply the same rule except that DCE forms an equilateral triangle.
Bernt Wahl mentions this fractal as a Kochawave in his Fractal Explorer.
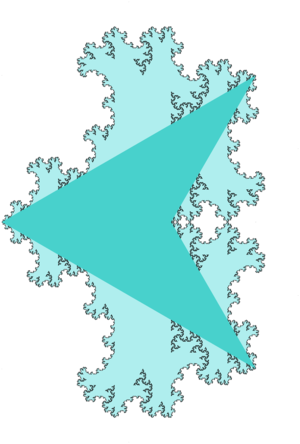
Arranging three copies pointing inwards around a triangle gives a fractal with empty area.
The previous fractal can be obtained by starting with an equilateral triangle and iteratively replacing each triangle by 3 copies scaled by a factor and one copy scaled by a factor .
The first substitution is rendered here with color blue.
The area after steps is the area of the original triangle, hence the limiting figure has empty area.
The Hausdorff dimension is approximately ().
Tiles
This tile is obtained by arranging three copies of the wave curve around an equilateral triangle.
Its area is twice the area of the original triangle.
It is possible to tessellate the plane by copies in one size.
This tile is obtained by arranging four copies of the wave curve around a rhomboid as depicted on the left.
It is possible to tessellate the plane by copies of sizes for .
It is possible to tessellate the plane by copies of sizes for .
This tile is obtained by arranging four copies of the wave curve around a dart as depicted on the left.
It is possible to tessellate the plane by copies of sizes for .
It is possible to tessellate the plane by copies of sizes for .
This tile is obtained by arranging two copies of the wave curve head to tail.
This tile can be obtained by arranging copies of the rhomboid tile scaled by a factor for .
It is possible to tessellate the plane by copies in one size.
This tile is obtained by arranging two symmetrical copies of the wave curve.
This tile can be obtained by arranging copies of the dart tile scaled by a factor for .
It is possible to tessellate the plane by copies in one size.
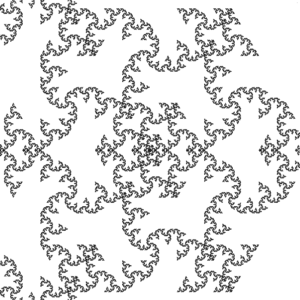
Tessellations
This tiling is periodic.
This tiling has scale symmetry.
This tiling has scale symmetry.
This tiling is periodic.
This tiling is periodic.