CERN Accelerating science

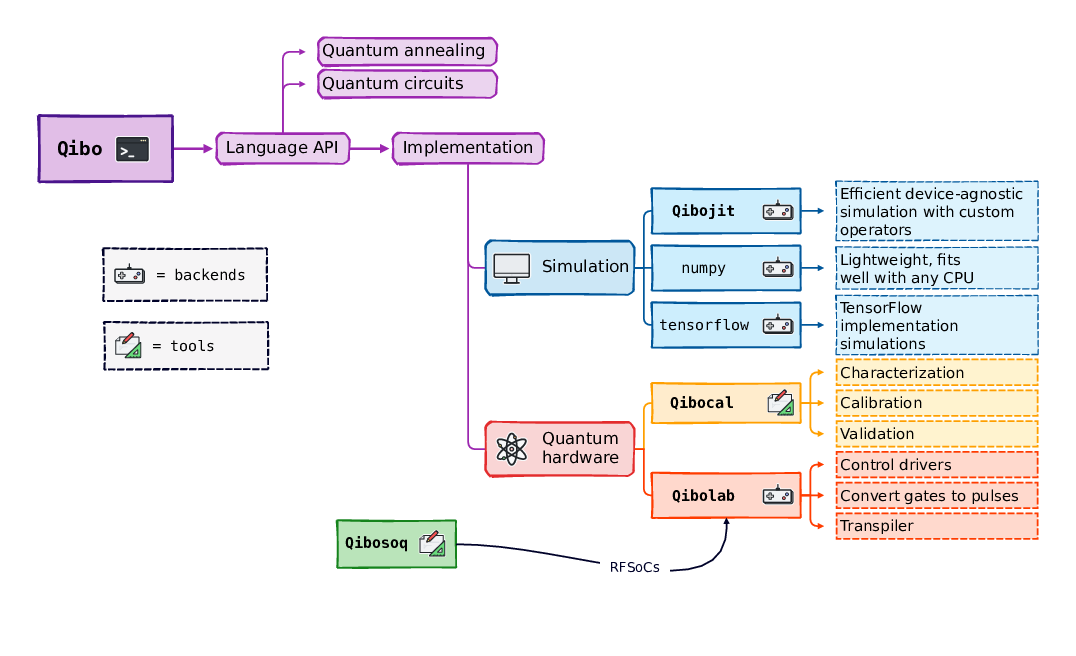Schematic overview of \Qibo software components, including backends and tools, for release \texttt{0.2.0}.

Schematic description of the \texttt{qibojit} backend features.

Basic setup of a self-hosted QPU. The host computer running \Qibolab communicates with the different electronics used to control a QPU.

Hierarchy of objects inside the \texttt{Platform}.

Benchmark of the performance of the built-in \Qibolab transpilers (routing pass) evaluated considering the CNOT overhead. Circuits of different types have been considered in order to test the transpilers on both structured and unstructured circuits. In particular we have considered a five qubits QFT circuit and random circuits with 5, 20 and 100 gates. The results for random circuits have been averaged over 50 circuits.

Execution time of different qubit calibration routines on various electronics. On the left side we show the absolute times in seconds for each experiment. The ideal time (black bar) shows the minimum time the qubit needs to be affected in each experiment. On the right side we calculate the ratio between actual execution time and ideal time. Real-time sweepers are used, if supported by the control device, in all cases except the \textit{Ramsey detuned} and \textit{Standard RB} experiments.

Scaling of execution time as a function of the number of points in a sweep. Bottom plots show the ratio between real execution on different instruments and minimum ideal time. Real-time sweepers are used in all cases, except the last \textit{Circuits} plot where we use the standard RB experiment to generate a given number of random circuits to execute.

Scaling of execution time as a function of the number of points in a sweep. Bottom plots show the ratio between real execution on different instruments and minimum ideal time. Real-time sweepers are used in all cases, except the last \textit{Circuits} plot where we use the standard RB experiment to generate a given number of random circuits to execute.

Scaling of execution time as a function of the number of points in a sweep. Bottom plots show the ratio between real execution on different instruments and minimum ideal time. Real-time sweepers are used in all cases, except the last \textit{Circuits} plot where we use the standard RB experiment to generate a given number of random circuits to execute.

Scaling of execution time as a function of the number of points in a sweep. Bottom plots show the ratio between real execution on different instruments and minimum ideal time. Real-time sweepers are used in all cases, except the last \textit{Circuits} plot where we use the standard RB experiment to generate a given number of random circuits to execute.

Scaling of execution time as a function of the number of points in a sweep. Bottom plots show the ratio between real execution on different instruments and minimum ideal time. Real-time sweepers are used in all cases, except the last \textit{Circuits} plot where we use the standard RB experiment to generate a given number of random circuits to execute.

Scaling of execution time as a function of the number of points in a sweep. Bottom plots show the ratio between real execution on different instruments and minimum ideal time. Real-time sweepers are used in all cases, except the last \textit{Circuits} plot where we use the standard RB experiment to generate a given number of random circuits to execute.

Results of single-qubit randomized benchmarking experiment implemented in \Qibo\ and executed with \Qibocal\, on a $5$-qubit IQM chip controlled by \Qibolab's Zurich Instruments drivers. Average relative frequency (survival probability) of classified $0$s of $128$ single-shot measurements (orange) for random sequences of single-qubit Clifford gates of different length and mean over $256$ random sequences (blue). The exponential fit is described by $m \mapsto 0.38(2)\cdot 0.9971(3)^m + 0.55(2)$. This corresponds to an average gate fidelity of $0.9986(2)$ and a $\pi/2$-pulse fidelity of $0.9992(1)$. Errors are the standard deviation of $1000$ `semi-parametric' bootstrapping samples (of binomial random variables with parameter drawn from the empirically observed distribution of relative frequencies).

Results of bare (yellow) and mitigated (red) CHSH values from an experiment on two qubits on a 5-qubit QuantWare chip controlled via \Qibolab's Qblox drivers. Readout error mitigation significantly enhances the results of the CHSH inequality, bringing it past the classical bound (blue line). The initial entangled state prepared for this experiment is $(\ket{01}-\ket{10})/\sqrt{2}$. The significant improvement produced by readout error mitigation hints that readout error dominates in the deterioration of the experimental results.

Estimates of $N_{\rm data}=50$ points of the $u$-quark PDF using the 1-qubit device controlled by the RFSoC. The target values (black line) are compared with the estimates obtained with the qubit. The solid orange line and the confidence intervals are calculated by repeating $N_{\rm runs}=50$ times the estimations with the trained model and then calculating means and standard deviation of the mean of the $N_{\rm runs}$ predictions. In particular, the two confidence intervals are computed using $1\sigma$ and $2 \sigma$ errors.