Overcoming exponential scaling with system size in Trotter-Suzuki implementations of constrained Hamiltonians: 2+1 U(1) lattice gauge theories
- Grabowska, Dorota M. et al
- arXiv:2208.03333CERN-TH-2022-133
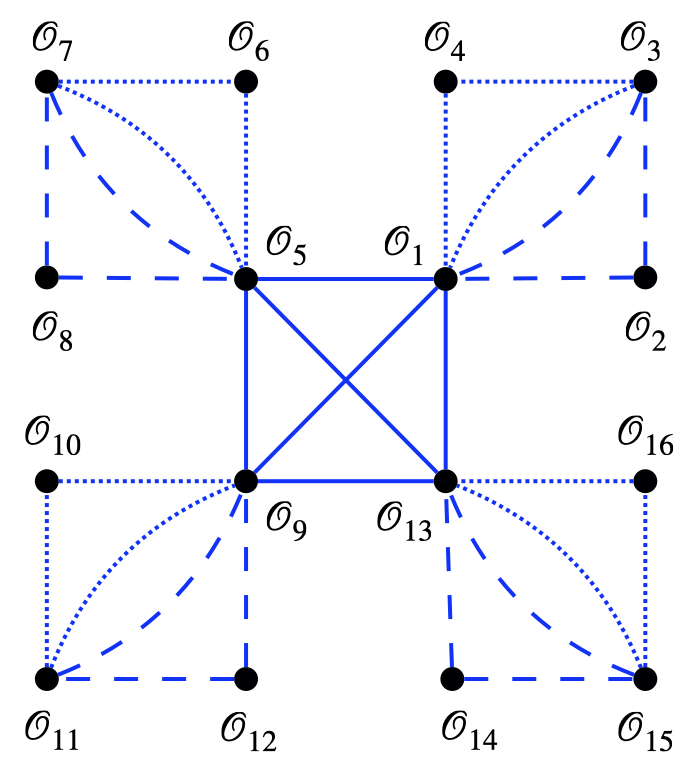
 | : Degree of coupling in the original basis. The system has maximum DoC, resulting in circuit depths that are exponential in the volume. |